INTERNAL DIMENSIONS OF THE GREAT PYRAMID
In 1865 Piazzi Smyth spent four months measuring the great pyramid of Giza. Smyth published his results in Our Inheritance in the Great Pyramid (1880). Flinders Petrie spent two winters between 1880 and 1882 measuring the pyramid. One of the express purposes of Petrie's study was to determine the accuracy of Smyth's findings. Petrie published his results in The Pyramids and Temples of Gizeh (1883). Both studies involved precise measurements and many of their findings are still relied upon today.
Smyth measured the pyramid in inches but he believed the pyramid was built in what he referred to as pyramid inches, 1.001 times longer than the modern inch. Smyth believed that the builders of the pyramid were aware of the size of the earth and used a measure that reflected this knowledge. The polar diameter of the earth is 500,500,000 inches. Given an inch 1.001 times longer than the modern inch, the polar diameter of the earth would be an even 500,000,000 inches. Petrie also measured the pyramid in inches, but he believed the pyramid was built in Egyptian cubits equal to 20.62 ± .005 modern inches. Today most Egyptologists and other researchers of ancient Egypt agree with Petrie that a cubit of 20.62 inches was used to build the great pyramid. Modern researchers also believe that the cubit was further divided into seven palms of four digits each, or 28 digits per cubit.
The antechamber in the great pyramid is 7.236 cubits (149.3 inches) high and 5.64 cubits (116.3 inches) long. The upper width of the chamber is 88 digits or 22 palms or 3.14 cubits. Granite wainscots four palms wide line the lower portion of the east and west walls of the chamber, making the floor and lower portion of the chamber two cubits wide. Three vertical slots one cubit wide are carved from the top of the wainscots to the floor. These slots held stones blocking entrance to the king's chamber. Three semicircular hollows were carved into the top of the wainscot on the west side of the chamber to hold beams for lowering blocking stones into place. The top of the wainscot on the east side of the antechamber is flat, five cubits (103.2 inches) above the floor of the chamber. The flooring block at the northern end of the antechamber is made of limestone. All of the other flooring blocks in the antechamber are made of granite and the granite portion of the floor is also five cubits (103.2 inches) long.
Smyth and Petrie both observed that the length of the granite portion of the floor of the chamber was the same as the height of the east wainscot above the floor of the chamber. Although there are slight variations in the height of the wainscot above the floor, possibly due to settling of the wainscot or the floor, Smyth and Petrie both believed that the equal length of the granite portion of the floor confirmed that the mean height of 103.2 inches was the intended height of the wainscot. 103.2 inches is equal to five ancient Egyptian cubits.
Smyth believed that the equal measures of 103.03 pyramid inches for the height of the east wainscot and the granite portion of the floor was intended to draw attention to the area of a square with sides of 103.03 inches (103.032 = 10615.18 inches). A circle with a diameter of 116.26 inches (the length of the antechamber) has an area of 10615.18 inches and a circumference of 365.24 inches. Smyth believed that the length of the of the antechamber was intended to be taken as the diameter of a circle with the same area as the square area of the east wainscot above the granite portion of the floor, demonstrating that the pyramid was built in inches and that the builders were aware of the precise length of the solar year. Although Smyth did not consider these dimensions in cubits, the height of the wainscot above the length of the granite portion of the floor gives a square area of 25 cubits. A circle with an area of 25 cubits has a diameter of 5.64 cubits (116.3 inches), equal to the length of the chamber.
Petrie rejected Smyth’s theory that the length of the antechamber was an expression of the diameter of a circle, in favor of his own theory that the square of the length of the antechamber was equal to 32 square cubits. This equates to 5.657 cubits or 116.7 inches for the length of the chamber. Petrie acknowledged that this is slightly longer than the mean length of the chamber, but it is within the absolute range of measures for the length of the chamber that he obtained from various heights in the chamber. Petrie believed the square root of 32 was the intended length of the chamber because many of the other dimensions of the chamber produce square areas that are whole numbers of cubits. The square of the wainscot height is 25 cubits. The square of the granite portion of the floor is 25 cubits. The upper height of the chamber (above the east wainscot) is 2.236 cubits. 2.236 is the square root of five so the square of the upper height of the chamber is five cubits. The square of the width of the floor is four cubits and the square of the width of the lower portion of the chamber is four cubits. The mean width of the portion of the chamber above the wainscots is 65 inches, or 3.15 cubits. Petrie believed that the intended width of the chamber above the wainscots was 3.16 cubits, which is also within the range of measures he obtained along the length of the chamber. The square area of an upper width of 3.16 cubits is 10 cubits.
The horizontal passage system that leads to the king's chamber begins at what is known as the great step, just north of the southern wall of the grand gallery. The edge of this step is at the north-south midline of the pyramid. Petrie gives the following measures in inches for the lengths of the blocks, the passages and the antechamber, from the edge of the great step to the king’s chamber:
| Edge of step |
-61.3 |
| South wall of gallery |
0.0 |
| North wall of antechamber |
52.02 |
| Joint, granite begins |
64.9 |
| Joint of floor |
112.15 |
| South wall of antechamber |
168.10 |
| Joint of floor |
198.41 |
| North wall of kings chamber |
269.04 |
| South wall of kings chamber |
475.36 |
These lengths generally convert to whole numbers of ancient Egyptian digits and all of the numbers in the diagram below are in digits unless otherwise specified. The flooring block that begins at the south wall of the gallery and ends in the antechamber is 64.9 inches long. This converts to 88 digits or three cubits and one palm. The same reasoning that Smyth and Petrie applied when they concluded that the height of the east wainscot was confirmed by the length of the granite portion of the floor should also be applied to the upper width of the chamber.
The mean upper width of the chamber is 65 inches. This is within 1/10th of an inch of the 64.9 inch length of the limestone flooring block. 64.9 inches is also an even number of 88 ancient Egyptian digits, unlike the mean width of 65 inches. 88 digits is 3.14 cubits which is also an accurate expression of π. This supports Smyth’s theory that the length of the chamber was intended to express the diameter of a circle, because the length of the chamber times the upper width of the chamber is equal to the circumference of a circle with a diameter equal to the length of the chamber.
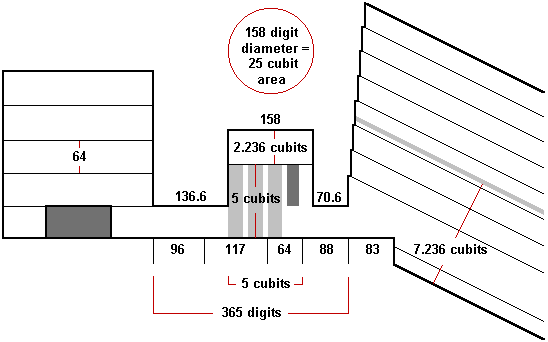
The first granite flooring block is 47.25 inches long (112.15 - 64.9 = 47.25). This converts to 64 digits or 16 palms. This is the same length as the height of the courses of the King’s chamber and the same length as the transverse height of the descending and ascending passages in the pyramid. The width of the floor of the antechamber is 14 palms (two cubits), the same as the width of the descending and ascending passages. As a result, the 60 palm perimeter of this block is the same as the perimeters of the ascending and descending passages (16 × 2 plus 14 × 2 equals 60). The perimeter of this block minus the length of the block is 44 palms or 6.2857 cubits. This is also an accurate expression of 2π (3.14 × 2 = 6.28). The second granite flooring block is 86.25 inches long (198.41 - 112.15 = 86.25). This converts to 117 digits or 4.18 cubits. This is 1/100th of the slant edge length of 418 cubits from the corner of the pyramid at ground level to the apex of the pyramid. 4.18 also closely approximates 4/3π.
The 116.3 inch length of the chamber converts to 158 digits. The length from the south wall of the grand gallery to the north wall of the antechamber is 52.02 inches. This converts to 70.6 digits. The upper height of the chamber is 2.236 cubits. The 70.6 digit length of the antechamber passage times 2.236 is 158 digits (the length of the chamber). The 7.236 cubit total height of the antechamber is also the same as the transverse height of the groove that was carved in the third overlap of the grand gallery.
The length of the king’s chamber from the north wall to the south wall is 206.32 inches (269.04 - 475.36 = 206.32). This converts to 10 cubits. The length from the great step at the midline of the pyramid to the north wall of the king’s chamber is 330.34 inches (269.04 + 61.3 = 330.34). This converts to 16 cubits. The length from edge of the great step to the south wall of the king’s chamber is 536.66 inches (475.36 + 61.3 = 536.66). This converts to 26 cubits.
The length from the south wall of the grand gallery to the north wall of the king’s chamber is 269.04 inches. This converts to 365.24 digits (269.04 ÷ 20.625 × 28 = 365.24). Petrie’s main objection to Smyth’s conclusion that the length of the antechamber was a diameter intended to give a circumference of 365.24 inches, was that the ancient Egyptians were not known to use inches. This objection does not apply to the length of 365.24 ancient Egyptian digits from the south wall of the grand gallery to the north wall of the king’s chamber.
In the ancient world, cubits were equal to one and a half feet. With a length of 20.625 inches for the ancient Egyptian cubit, the ancient Egyptian foot was equal to 13.75 inches or 1.1458 English feet (20.625 ÷ 1.5 = 13.75 inches and 13.75 ÷ 12 = 1.1458 feet). The equatorial circumference of the earth is 24,901 miles. The equatorial diameter is 7926.2345 miles:
24,901 ÷ π |
= |
7926.2345 miles |
7926.2345 × 5280 |
= |
41850518 English feet |
41850518 × 12 |
= |
502206216 English inches |
502206216 ÷ 20.625 |
= |
24349392 cubits |
24349392 × 1.5 |
= |
36524088 Egyptian feet |
The number of ancient Egyptian feet in the equatorial diameter of the earth is equal to the number of days in 100,000 solar years. This supports the conclusion that the length of 365.24 digits between the south wall of the grand gallery and the north wall of the king’s chamber was intentional and also supports the conclusion that an ancient awareness of the size of the earth and the length of the solar year was incorporated into the measurement unit of the ancient Egyptian foot.
The floor of the grand gallery from the north wall to
the great step is 1815.5 inches, or 88 cubits, or 28 times the width of the
antechamber. The floor is two cubits wide, but the base of the gallery is
four cubits wide. Blocks one cubit wide and one cubit high line the length
of the gallery on both sides of the base. The walls are comprised of seven
overlapping courses. Each successive overlap is one palm narrower on each
side, so the ceiling of the gallery is also two cubits wide. The width of
the gallery at the third overlap is 22 palms or 3.14 cubits, the same as the
upper width of the antechamber. The groove in the third overlap is half way
between the floor and the ceiling of the gallery. The diagram below shows
the south wall of the gallery, the great step and the entrance to the antechamber
passage.
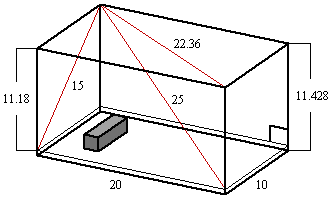
The king’s chamber is 10 cubits wide and 20 cubits long. All four walls of the king's chamber consist of five courses of equal height. Each of the courses is 64 digits, or 16 palms high, so the total height of the chamber from the base of the walls to the ceiling is 320 digits or 80 palms or 11.428 cubits. Expressing all of the dimensions of the chamber in palms, the width is 70 palms, the height is 80 palms and the length is 140 palms, the proportions being 1 : 1 1/7 : 2. The length plus the height is 3 1/7, or 3.1428. In cubits, the length plus the height of the chamber (11.428 + 20) is 31.428 cubits. 31.428 cubits divided by the width of the chamber (10 cubits) equals 3.1428. The perimeters of the long walls of the chamber are 440 palms (80 × 2 plus 140 × 2). Dividing the perimeter of the wall (440 palms) by the length of the wall (140 palms), also demonstrates π: 440/140 = 22/7 = 3.1428. Given the length of the king’s chamber as the diameter of a circle, the circumference of the circle is equal to the perimeter of the long walls of the chamber.
The granite floor of the king's chamber is not connected to the walls and the top of the floor blocks are seven digits (one quarter of a cubit) above the base of the first course of the walls of the chamber. Thus, the height of the chamber from the top of the floor blocks to the ceiling is 11.18 cubits (320 - 7 = 313 digits and 313 digits ÷ 28 = 11.18 cubits). The height of the upper portion of the antechamber is 2.236 cubits and the height of the wainscot is five cubits, so the total height of the antechamber is 2.236 + 5 cubits.
The height of the king’s chamber from the floor to the ceiling is 2.236 × 5 cubits = 11.18 cubits. 11.18 is the square root of 125. The diagonal length of the chamber is 22.36 cubits, twice the height. 22.36 is the square root of 500. The diagonal length of the short walls of the chamber is 15 cubits. The diagonal length from upper corner to opposite lower corner is 25 cubits. The right triangle formed by this diagonal, the short wall diagonal and the floor length forms a 3-4-5 right triangle (15-20-25). The volume of the chamber is 2,236 cubic cubits (20 × 10 × 11.18).
Petrie gives the following measures in inches for the mean dimensions of the coffer in the king’s chamber:
| Outside | Inside | |
| Length | 89.62 | 78.06 |
| Width | 38.50 | 26.81 |
| Height | 41.31 | 34.42 |
These measures convert to ancient Egyptian cubits as follows:
|
Outside |
Inside |
|
|
Length |
4.346 |
3.785 |
|
Width |
1.867 |
1.3 |
|
Height |
2 |
1.67 |
The outside volume of the coffer is 16.22 cubic cubits and the inside volume of the coffer is 8.22 cubic cubits. This leaves a difference of eight cubic cubits representing the solid bulk of the coffer. Thus the bulk of the coffer is equal to a solid cube with sides the same length as the height of the coffer. The diagonal of the inside floor of the coffer is four cubits (3.7852 + 1.32 = 42). This is twice the height of the coffer, just as the diagonal of the floor of the king’s chamber is twice the height of the chamber. The inside perimeter (3.785 × 2 plus 1.3 × 2) is 10.17 cubits, equal to the circumference of a circle with a radius of φ (1.618 cubits).
This circle has an area of 8.22 cubits, equal to the inside volume of the coffer. Michael Saunders pointed out that a sphere with a diameter of π (3.14 cubits) has a volume of 16.22 cubic cubits, equal to the outside volume of the coffer. A sphere with a diameter of 16.22 cubits has a volume of 2,236 cubic cubits, equal to the volume of the king’s chamber. The radius of this sphere is 8.11 cubits, equal to the area of the outside base of the coffer.
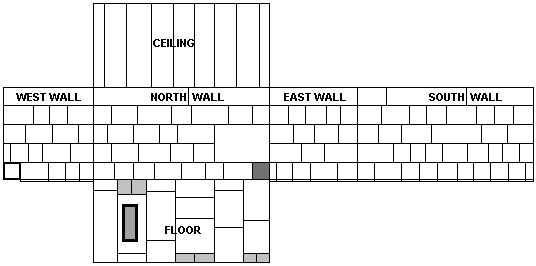
There are a total of 100 blocks in all four walls of the king’s chamber. The dark square in the north wall is the entrance passageway into the chamber. The east wall has 18 blocks. The north wall has 27 blocks and the south wall has 36 blocks. The ceiling has nine blocks. The floor originally had 18 blocks in six courses, although the floor presently has 21 blocks. The three blocks in the lightly shaded areas were pulled out by early explorers and have been replaced with six blocks.
The joints between the blocks in the walls of the king's chamber are 1/50th of an inch wide. In the 1970's and 80's J. P. Lepre conducted a 15 year study of the pyramids of Egypt, concentrating on the Great Pyramid. Lepre found that the southernmost block on the first course of the west wall was the one exception to the tightly fitted joints in the walls of the king's chamber. The joints around this block are 1/16th to 1/4th of an inch wide. Lepre also found that the base of this block is at the level of the floor blocks of the chamber, rather than a quarter of a cubit below the level of the floor, like all the other blocks on the first course of the walls of the chamber. Based on this information, Lepre proposed that this block may be blocking another passageway into or out of the king's chamber.
The west wall has 19 blocks, including the block that Lepre suggests is blocking a passageway. If Lepre is correct then the west wall would have 18 blocks with the passageway open and the number of blocks in all six surfaces of the king’s chamber would be multiples of nine: South wall - 9 × 4; north wall - 9 × 3; east wall, west wall and floor - 9 × 2; ceiling - 9. This is a total of 126 blocks in the chamber and 99 blocks in all four walls. The ratio between the total number of blocks in the chamber and the number of blocks in the walls is 126/99.
This reduces to 14/11 or 4/π, the same ratio that is found in the external slope of the pyramid.