APPENDIX TWO
USING THE SUMERIAN PENDULUM

The method used by the Sumerians to set their own basic unit of length, the double kush, followed the same general rules as those employed by the Megalithic peoples of the far west of Europe. The only difference lay in the numbers used.

Sumerians relied on a 360° geometry, of the type we still use today.

Because of this their starting point was to divide the horizon into 360 equal units. The mathematical trick used to short-circuit this procedure that was itemized in Appendix One does not apply in this case. It is possible that the Sumerians devised their own method of making the initial procedure quicker, but in any case theirs was a metal-using culture and one that would therefore not have needed to repeat the procedure of defining the linear unit all that often.

They could have created a fairly accurate standard rod. Establishing the necessary 1/360th of the horizon by trial and error would have taken time, but it is quite possible to achieve with a high degree of accuracy.

The procedures used in the preceding Appendix are now followed. The braced frame would be equal to a gap of 1/360th of the horizon but Venus would be tracked in exactly the same way.

The desired number of swings in this case is 240, which is the same as 240 seconds, a period of time known to the Sumerians as a 'gesh'.

A pendulum that swung 240 times during the passage of Venus through the braced frame would be 99.88 cm in length, a linear length that conforms to that discovered on the statues of Gudea from Lagesh in Iraq. This unit of length was known to the Sumerians as the double kush.

It has to be noted that the pendulum in question is not strictly speaking a seconds pendulum of the sort that was popularly used from the seventeenth to the nineteenth century.

Because the object being tracked is Venus, which is moving independently against the backdrop of stars, the time taken for each beat of the pendulum is slightly longer than a second (1.002 seconds). This stands as part of the proof that the Sumerians did use this system to define their linear unit.

They fully understood that there were 43,200 seconds in a day (to us there are twice this number because we use a twenty-four-hour day instead of the Sumerian twelve-hour day) but there is no absolutely reliable way of defining the true second of time by observing the sky and swinging a pendulum. This could only be achieved by tracking the average movement of the Sun in the same way Venus is used in this exercise.

However, because of the Earth's own orbital characteristics, the Sun does appear to move at a constant speed across the sky.

There are only a few days each year on which the experiment using the Sun would work perfectly and the Sumerians could not have known which days would have been appropriate. In addition, the Sun is very much more difficult, and potentially dangerous, to track in this way.

Similarly, if they had used a star instead of the planet Venus, the pendulum would still not have been a true seconds pendulum . The reason for this is that the sidereal day (a day that is measured by a star passing from one point in the sky back to that point again) is shorter than a solar day (a day that is measured by the Sun passing from one position in the sky back to that point again).

A seconds pendulum created by tracking a star would actually give a time reading of 0.997 seconds and lead to a pendulum length close to 99.3cm.

We remain convinced that both the Megalithic culture and that of the Sumerians were simply following instructions that had been given to them by another agency. In the case of the Sumerians the use of Venus for setting their pendulum, and therefore their chosen unit of length, resulted in a series of measurements that were truly integrated with the Earth.

As we have shown, the Sumerian double mana unit of mass divides into the mass of the Earth 6,000,000,000,000,000,000,000,000 times, which would not have been the case with a shorter pendulum and therefore a lighter unit of mass.

True, the achieved second of time was slightly at odds with the genuine second of time, but the Sumerians, lacking accurate clocks, could not have been aware of this fact.

In fact the discrepancy is so small it couldn't have been measured until the last century or so.

Back to Contents

APPENDIX THREE
THE MESSAGE IN DETAIL

The message that we have detected is present in recurring number sequences that are, strangely, often round numbers.

We started to realize that something highly unusual was happening when we discovered that the Megalithic system of geometry worked on the Moon and the Sun as well as the Earth.

Looking into issues concerning the Moon we were immediately reminded of the strange coincidence that the Moon and the Sun appear to be the same size in Earth's skies, leading to the phenomenon we call a total eclipse. Still stranger is the fact that the relation is so numerically neat with the Moon being 400 times smaller than the Sun and 400 times closer to the Earth at the point of a total eclipse.

On its own this could be a bizarre coincidence, but because of what follows we believe that it is the 'headline' to a message built into the Moon 4.6 billion years ago.
 

The Megalithic system

The Megalithic system of geometry is based on 366° to a circle, sixty minutes to a degree and six seconds to a minute.

This sequence produces a second of arc on the Earth's polar circumference that is 366 Megalithic Yards long, the linear measure of the Megalithic builders as identified by Alexander Thom.

As a cross-reference we had also discovered that the 4,000-year-old Minoan Foot is precisely equal to a 1,000^th part of a Megalithic second of arc.

We applied the principles of Megalithic geometry to all of the planets and moons in the solar system and found that it only produced round integer results for the Sun and the Moon. The Sun is very close to being a true sphere, certainly much more so than the Earth.

NASA quote the mean volumetric circumference as being 4,373,096 km, which we converted into Megalithic Yards and applied the 366 geometry.

Sun's circumference = 5,270,913,968 MY

One degree = 14,401,404 MY

 

One minute = 240,023 MY

 

One second = 40,003.8 MY

The fit is 99.99 per cent accurate to 40,000 and given that this is based on a best estimate of the mean circumference it has to be considered bang on.

Like the Sun, the Moon is quite close to being a sphere.

NASA gives the mean volumetric circumference of 10,914.5 km, which produces the following result:

Moon's circumference = 13155300 MY

 

One degree = 35943 MY

One minute = 599 MY

One second = 99.83 MY

If we use the equatorial radius the result is 99.9 MY per second of lunar arc.

Either way, this is as close to 100 MY as makes no difference, given the irregular surface of the Moon and the small variation in Thom's definition of the Megalithic Yard of +/- 0.061cm.

It could have been possible for people many thousands of years ago to create a system of geometry that produces round integers for two celestial objects such as the Earth and the Sun, but it would seem impossible to achieve such a feat for three bodies.

It therefore appears that the Moon was designed using units derived from the physical dimensions of the Sun and the Earth.
 

The Earth-Moon relationship

The duration of the Moon's orbit (sidereal - fixed star to fixed star) is 27.322 Earth days (27.396 rotations of the Earth). This number is extraordinarily close to the size relationship of the Moon to the Earth, being 27.31 per cent of the Earth's size.

The Earth currently turns on its axis 366.259 times for each orbit around the Sun. This number is extraordinarily close to the size relationship of the Earth to the Moon, being 366.175 per cent larger than the Moon.

There is no reason why these numbers should repeat in this way:

Earth turns per cent size of polar per orbit circumference

• Earth 366.259 27.31

• Moon 27.396 366.175

It is also a consequence of the above that the Moon makes 366 orbits of the Earth in 10,000 Earth days.

The size of the Sun, Earth and Moon have been fixed for billions of years so their size ratios have not changed. But the orbital characteristics of the Earth and the Moon have changed constantly.

When the Moon was much closer to the Earth than it is now, its orbit was much shorter and the Earth day was also shorter, leading to perhaps as much as 600 days to the Earth year.

The Earth's own orbit around the Sun remains essentially unchanged. It is only the time it takes to spin on its own axis that alters.

The close number association between the size ratios of the Sun, Moon and Earth, and the orbital characteristics of the Moon, together with the present length of the Earth day, are only applicable to the time that humans have been fully form ed. These relationships were not present in the distant past and they will disappear in the distant future.

The number sequences which alerted us to the 'message' are clearly meant for the present period.
 

The Metric System

Orbital characteristics and size relationships are physical factors and any correlations are real - no matter what units of measurement are employed.

No one knows the origin of the Megalithic system but the origin of the metric system is fully documented. Whilst it did have a near identical precursor in the Sumerian system of m ore than 4,000 years earlier, the metric system is known to have been developed from measuring the polar circumference of the Earth alone.

It was designed so that there should be 40,000km in one Earth circumference. The equator is a little longer than the polar circumference but basically the Earth turns through this distance each day.

The Moon turns through an unimpressive sounding 10,920.8 kilometers every 27.3217 days. This converts to 400 km per Earth day - to an accuracy greater than 99.9 per cent. Again this is a factor that only exists in the human period of existence.

The number 400 is already central to hum an appreciation of the Moon because it is 400 times closer to us than the Sun, and it is 400 times smaller. The use of 400 kilometers per current Earth day could be a message that the architect of the Moon knew we would use kilometers and mean solar days.

Metric units apart, the Moon is turning at a rate that is almost exactly one per cent of the Earth's rotation. Or to reverse the factor, the Earth is turning 100 times as fast as the Moon.

All curiously round values!

To add to the idea that this is a deliberate piece of metric design, the Moon is also travelling on its journey around the Earth at a steady rate of one kilometer per second! This speed varies a little as it travels but does not drop below 0.964km per second and does not exceed 1.076km per second.

And there is something else very special about the kilometer as regards the Moon. To understand it we need to realize that there are 109.2 Earth diameters across the Sun's diameter.

There are also 109.2 Sun diameters between the Earth and the Sun at its furthest point of orbit. The circumference of the Moon is 109.2 x 100 kilometers.

Is that not odd in the extreme?

One way of looking at the association between these ratios and numbers can be seen in the diagram in figure 16. There are many factors here that should bear no relationship with each other at all.

Taken in isolation, any one of these strange associations might be considered to be a coincidence but there comes a time, however, when coincidences become so frequent that something else must be at work.
 

Figure 16
 

Back to Contents

APPENDIX FOUR
THE MECHANICS OF ECLIPSES

The awesome sight of a black shadow gradually crossing the face of the Moon still captivates most people, even though we now live in an age when we not only know what causes the phenomenon but can predict exactly when it is likely to happen.

Early cultures did not know either and must have seriously thought, for a few minutes at least, that the world was coming to an end.

Back in the 1960s the astronomer Gerald Hawkins suggested that at least one of the functions of the structure at Stonehenge, Salisbury Plain, England, was to predict the occurrence of eclipses.

Hawkins had carefully studied the ancient monument, parts of which date back five thousand years, and subjected his data to a massive number- crunching computer. He came to the conclusion that the Aubrey Holes, a series of fifty-six chalk-filled pits around the standing stones at Stonehenge, represented a sophisticated device for predicting both solar and lunar eclipses.46

Clay tablets discovered in what is now Iraq and dating back to the Sumerian period, which commenced around 3000 BC, indicate that people in the region were doing their best to predict eclipses. And there isn't any doubt at all that the Babylonians who followed the Sumerians were competent at accurately working out when the face of the Sun or Moon would darken.

The ancient Chinese, Indians, Egyptians, American cultures and many other societies worked hard to develop an understanding of rudimentary astronomy for the purpose of eclipse prediction.

This single effort certainly caused humanity to significantly improve its naked-eye astronomy and its understanding of mathematics. There are good reasons why this should be the case and at the base of most of them is power. Any would-be ruler, secular or religious, who could predict when an eclipse was likely to take place was in a very strong position to manipulate the situation to his or her own ends.

To the average lay person, eclipses seem to be totally haphazard but this is not the case.

However, such is the complicated nature of the interplay of the Earth and the Sun that understanding eclipse patterns is far from easy. Once the pattern is cracked, its secrets could be passed from one ruler to another and a whole society could be alerted to a possible eclipse.

The prediction itself would have seemed to most people to be the most sophisticated sort of magic and when the king or holy man drove away the dark dragon that was trying to swallow the Sun or causing the Moon to bleed, his power would be ensured for a considerable period ahead.

What the ancients gradually discovered was that there were very definite patterns to the occurrence of all eclipses and that they were governed overall by a specific period of time that is known as the 'saros cycle'.

The word saros was first used by the astronomer Edmond Halley (1656-1742) and is supposed to have been derived from a Babylonian word. The saros cycle is 6,585.3 days in length (18 years, 11 days, 8 hours).

It represents the coming together of three distinct patterns. The first of these is the Synodic Month (new Moon to new Moon,) the second is the Draconian Month (node to node [see below for information on Moon's nodes]) and the third is the Anomalistic Month (perigee to perigee [see below for information on Moon's perigee]).

To within about two hours, 223 synodic months, 242 draconian months and 239 anomalistic months come out to the same period of time and it is at this point that any eclipse will repeat itself. The reason for this is that the solar system runs pretty much like a gearbox and, as with a gearbox, any pattern created now will sooner or later be repeated.

Although the saros cycle is very accurate, there are many such cycles running at the same time. All that can be deduced from the saros cycle is that if an eclipse occurs today, it will occur again in 6,585.3 days and will have a quite similar geometry. The system does fall down somewhat in that it splits a day and so future eclipses in any given cycle may not be fully visible from the same part of the globe.

Each saros cycle runs for around 1,200 years (around sixty-six repeat eclipses) until it expends itself. If the saros cycle commences near the South Pole it will extend itself gradually further north with each eclipse until it finally disappears at the North Pole. The same is true in reverse.

It would appear that the Babylonians understood the saros cycle, as did the Ancient Greeks.

So, according to Gerald Hawkins, did the builders of Stonehenge. Something akin to the saros cycle would have been useful to ancient peoples because if the next eclipse in any given series was less impressive than the last, it would still have been predicted, and it was just as likely to be m ore im pressive as less so. (Better by far to turn the tribes out for a less-than-spectacular display than to miss what could be a super show!)

Our own previous research demonstrates that following the saros cycle was actually very easy for the Megalithic people, who were the builders of Stonehenge and thousands of other such monuments. The ritual year of the Megalithic cultures was 366 days in length.

This meant that the saros cycle to them was just two days short of eighteen years in length. The two days didn't really matter because solar eclipses can only occur at the new Moon and lunar eclipses at the full Moon.

In other words, just a couple of days short of eighteen years after a particular eclipse, the next full or new Moon would be certain to bring another.

Even today we don't take solar eclipses for granted. A major eclipse, such as the one that was visible in northern Europe on August 11th 1999 is treated as a time of celebration and is now revered for its sheer beauty, rather than being feared as was surely the case even not so long ago. The face of the Sun gradually begins to blacken as the Moon passes between it and the Earth.

If it is a full eclipse the Sun's disc will be covered at what is known as totality.

At totality, all that is perceptible is the faint glow from the corona of the Sun. Soon after, the shadow begins to move away and a spectacular shaft of light breaks out, forming what is known as the diamond ring effect. The phenomenon is just as impressive now as it must have looked from Babylon or Stonehenge.

It might surprise readers to learn that no matter where our astronauts or cosmonauts travel in the future within our solar system , they will never stand on the surface of any other planet and watch a total eclipse.

They are simply not possible anywhere else and only occur as a legacy of a series of breathtaking, apparent coincidences.

The fit of the Moon's disc across the face of the Sun during a total eclipse is not 'near' - it is 'exact '- and this fact should be the greatest sense of wonder to anyone viewing such an event because it is very unlikely.

No other planet has a moon anywhere near big enough or orbiting at the right distance to fully, but not too fully, eclipse the Sun. There are two basic sorts of eclipse, and then subcategories within the two types. The most impressive form of eclipse is known as a solar eclipse.

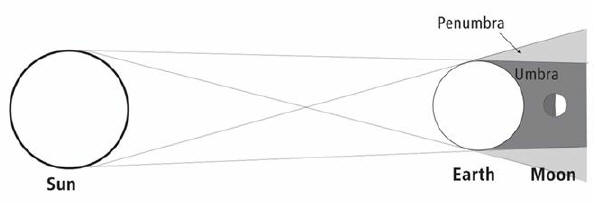
The drawing below shows what is actually happening when a solar eclipse takes place.

Figure 17
 

When the Moon stands directly between the Earth and the Sun, a total eclipse is possible but totality only occurs across a relatively small area of the Earth's surface and follows a curve known as the Path of Totality.

In this example, which is a 'total eclipse', to a proportion of those people living along the path of totality, the disc of the Sun will be blotted out completely. Whilst totality is achieved, all that can be seen is the sun's corona (the halo of bright matter that is constantly being thrown off by the Sun).

The larger shadow is called the penumbra and people beneath this will see a partial eclipse. There is another form of solar eclipse that can never be total and this is known as an annular eclipse.

The Moon is 1/400^th part the size of the Sun and it stands at 1/400th the distance between the Earth and the Sun, but not always exactly.

The Moon's orbit around the Earth is not circular but elliptical. This means that sometimes the Moon is slightly closer to the Earth than it is at other times. If a solar eclipse takes place when the Moon is furthest from the Earth, the Moon's disc looks smaller and can never totally blot out the Sun. Total eclipses of the Sun therefore happen when the Moon is on the part of its orbit that brings it closest to the Earth.

When the Moon is closest to Earth it is said to be at perigee and when it is furthest away it is at apogee.

Solar eclipses can only take place when the Moon stands between the Earth and the Sun and this is the short period on each lunar cycle known as 'new Moon'. (The time of the lunar month when no part of the Moon is visible from Earth.)
 

It might be thought that because there is a new Moon each month, there should therefore be a solar eclipse each month but this is not the case.

The orbit of the Moon around the Earth does not follow the same angle as the orbit of the Earth around the Sun. If it did, every new Moon would indeed bring a solar eclipse. Rather it is tilted to the Earth's orbit (known as the ecliptic) by five degrees. Only when new Moon occurs at a point when the orbit of the Moon around the Earth crosses that of the Earth around the Sun, can a solar eclipse take place.

These points north and south of the ecliptic are called the Moon's nodes. This happens 'at least' twice each year and can produce a solar eclipse observable from somewhere on the Earth.

The second type of eclipse is not quite so impressive as a solar eclipse but it would have been fascinating to our ancient ancestors all the same. It is more common than a solar eclipse and is known as a lunar eclipse.

A lunar eclipse takes place when the shadow of the Earth comes between the Sun and the Moon. A lunar eclipse can only take place at the exact opposite time to a solar eclipse, at the time of the full Moon when the entire disc of the Moon is visible from Earth.

During a lunar eclipse the face of the Moon does not disappear altogether. Rather it is darkened and, under some circum stances, it appears to turn a deep red.

Such lunar eclipses were seen by many ancient cultures as terrible harbingers of disaster and were probably feared as much as solar eclipses.
 

Figure 18
 

The path taken by the Earth around the Sun is not the same as that taken by the Moon around the Earth. There is a 5° difference.

Because of this, total eclipses can only happen when new Moons fall on what is known as the node - that point at which the two orbits cross.
 

Figure 19
 

A lunar eclipse takes place when the Earth's shadow crosses the face of the Moon at the time of full Moon.

Once again the fact that the plane of the Earth's orbit around the Sun and that of the Moon around the Earth are not the same prevents every full Moon from being eclipsed.

Until we did some in-depth research we never realized just how unlikely or extraordinary a total eclipse actually was. It's all a matter of 'line of sight' as the diagram s below should make clear.

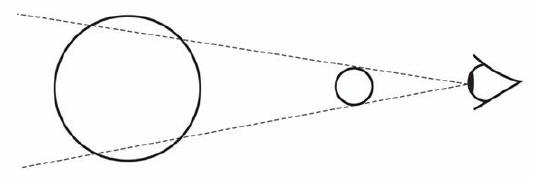
Isaac Asimov, the famed science-fiction guru, described this perfect visual alignment as being:

'The most unlikely coincidence imaginable'.

Figure 20
 

In this example the eye on the right looks past a small sphere to a much larger sphere.

The size of the spheres and the distance between them is such that because of the perspective to the viewer the small sphere will exactly cover the large sphere.

 

Figure 21
 

Now the small sphere is even smaller, but is at the same distance from the eye of the observer. Under these circumstances the eye will also see part of the larger sphere.

Finally, if we keep the spheres the same as in the last example, but move the smaller one nearer to the eye of the observer we once again create a situation in which the small sphere appears to exactly cover the large one.

 

Figure 22
 

The sphere of the Sun is image almost exactly 400 times larger than that of the Moon.

This in itself might be considered nothing more than a strange but meaningless coincidence but we must stretch coincidence almost to breaking point when we realize that when the Moon is as close to the Earth as its orbit will bring it, it stands at 1/400th the distance between the Earth and the Sun.

Under these circumstances when it stands precisely between the observer and the Sun, the Moon 'must' exactly cover the disc of the Sun - it is a simple matter of perspective.

In the case of total eclipses we really are living in a tiny snapshot of the history of the Earth and the Moon. The Moon was very much closer to Earth at the beginning of the relationship, and by the time the two reach a situation of perfect stasis the Moon will be 1.6 times further away from the Earth than it presently is.

If we estimate the Moon to be 4 billion years in age and then accept the most common assessment that it will reach its furthest position from the Earth in 15 billion years (excluding the fact that the Sun will most certainly have gobbled up both Earth and Moon by then) the sum total of the Moon's journey from closest to furthest from the Earth is 19 billion years.

The Moon is a finite size, as to all intents and purposes is the Sun. There can only be a very short window of opportunity during which the disc of the Moon can cover that of the Sun, as seen from Earth, in the truly perfect way that it does right now.

That it has done so just at the time we have evolved into a sophisticated enough species to recognize and study the fact seem s almost incredible. It doesn't matter how much experts say 'It's just one of those things', it is still an example of one of the most unlikely coincidences imaginable.

It stands to reason that if the Moon were any larger or smaller than it is, total solar eclipses would not be possible at this time. A smaller Moon would have brought such phenomena in the very distant past, when the Moon was much closer to the Earth than it is now.

This brings us to a discussion of the dimensions of the Moon. In comparison with the size of its host planet the Moon is huge. Its circumference would only fit into that of the Earth 3.66 times. Another of the terrestrial type planets, Mars, has two moons, but they are tiny when set against Earth's Moon, which in terms of size might reasonably be termed a planetoid.

Even bearing in mind the vast size of the planetary super-giants, with their proliferation of moons, Earth's Moon is still the fifth largest in the whole solar system.

A close examination of Moon rock, brought back by both American astronauts and Soviet unmanned missions, shows that they are very similar to specific rocks on the Earth. Analysis of the rocks proves that they were created at the same distance from the Sun, so there is no longer any real doubt that the Earth and the Moon have a common origin.

Yet there is something very strange about the Moon that isn't easy to explain. Although it is 1/3rd as big as the Earth it has only 1/81st of the Earth's mass.

Had the Moon been composed of a representative sample of 'all' Earth's rocks and still been the size it is, it would have been much more massive. Conversely, if the Moon had exactly the same com position as the Earth and had exerted the gravitational pull it presently does, it would have been very much smaller in size.

These facts are discussed in greater detail elsewhere, but it is the weird composition of the Moon, which is comprised of very light, Earth-type rocks, that means it can be large enough to create a total solar eclipse and yet not rip the surface of the Earth to pieces with its gravitational pull every time it passes overhead.

In a Universe filled with incredible wonders, and one so big that it might as well be infinite as far as we are concerned, we are certain to stumble across what looks to us like outrageous coincidences.

Even conservative astronomers admit that total eclipses are very unlikely but still maintain such happenings must be a random chance event.

We beg to differ!

Back to Contents
 

APPENDIX FIVE
THE WORLD FROM A BARLEY SEED

The previous work we had undertaken for our book Civilization One had featured a number of ancient measuring systems.

None of these surprised us m ore than that created by the Sumerians, a culture that originated in what is presently known as Iraq at about the same time as the Megalithic culture was flourishing in Britain and France. Our ongoing work for the present book made us look again at some aspects of the Sumerian measuring system.

It could be that yet another part of the message left to us, indicating a deliberate intervention into the origin and progress of humanity, is encapsulated within the methods the Sumerians used to measure their world.

Out of a plethora of different linear lengths, weights and measures, it was possible for us to reconstruct the entire Sumerian system as we are sure it was originally meant to be. We have demonstrated how the Sumerians used a pendulum and the planet Venus in order to establish the basic unit of linear length, which was known as the double kush.

Existent statues of the Sumerian King Gudea demonstrate that the double kush was intended to measure 99.88cm. Units of volume and weight were derived from the double kush by creating a cube with sides of 1/10th of a double kush.

The amount of pure water held by such a cube represented the sila, which was the Sumerian unit for measuring volume. The weight of this water was known as the mana or mina and was the Sumerian unit for measuring m ass.

How we untangled all of this from the Sumerian records is explained in detail in our book Civilization One.⁴⁷

There seem ed to be no doubt that the double kush had indeed been created by way of a pendulum and observations of the planet Venus but it was not the only way the Sumerian system could be recreated. Everything in the system also relied on the size, shape and weight of a humble barley seed.

To the Sumerians a barley seed was known as a se.

Until our own investigations, many experts had believed that the use of the barley seed by the Sumerians for measuring purposes was probably an abstraction. It was generally considered that the Sumerians might originally have used such seeds (as was the case in ancient western Europe), but that as in the case of Europe the seeds ultimately came to be words representing sizes and weights that no longer related to barley seeds at all.

The Sumerians claimed that 360 barley seeds was the measure of the double kush, something that experts on the Sumerian culture actively deny or at best have totally ignored.

Our extensive investigations showed conclusively why this state of affairs had com e about. Experts had undoubtedly assumed that if the Sumerians had used barley seeds as tiny units of length, they must have laid the seeds end to end. It is likely to be for this reason that it is now generally considered that the seeds them selves eventually lost all contact with units of measure, because when they are laid end to end they make no sense at all.

However, we discovered that if the seeds were laid on their sides and front to back (as they may have been carefully strung on a necklace) they conformed absolutely to the Sumerian system.

We then went on to demonstrate, by practical experiment, that the Sumerians had also been quite correct in their estimation of the 'weight' of an average barley seed and we were staggered to discover that even modern barley seeds have almost exactly the same dimensions and weight as their Sumerian counterparts.

It has been possible to show that in terms of mass measurement the whole Sumerian system was irrevocably and seemingly quite deliberately tied to the overall mass of the Earth itself. We appreciate that this sounds absolutely absurd for such an early culture but when one sees the figures involved, there is no doubt about it.

According to Sumerian texts it was considered that there were 10,800 barley seeds to the unit of weight known as the 'mana'. The weight of water held in the double mana, assuming a double kush of 99.88 cm and a cube with sides of 1/10^th of this, would have been 996.4 grams.

The mass of the Earth is held to be 5.976 x 10²⁴ kg. If we divide this by .9964 in order to establish how m any double mana there are to the mass of the Earth, we arrive at 5.99759 x 10²⁴ double mana. This number is so close to 6 x 10²⁴ (99.99per cent) that this must surely have been the number intended.

Since there are 10.800 barley seeds to the mana and therefore 21,600 to the double mana it is possible to show that the mass of the Earth is equal to that of 1.296 x 10²⁹ barley seeds. This might not seem to be a particularly impressive number but it has some very important properties.

If we were to segment the Earth, as we might an orange, we would discover that each 1/360^th segment of the Earth has a mass equal to 3.6 x 10²⁶ barley seeds.

A further split of sixty brings us to 6 x 10²⁴ barley seeds and yet another split of sixty results in 1 x 10²³ barley seeds, which can be expressed fully as 100,000,000,000,000,000, 000,000.

The starting point of this exercise was an Earth mass of 6 x 10²⁴ double mana for the mass of the Earth, which would have been highly significant in Sumerian terms since there's was a sexagesimal (sixty base) system.

For all the reasons explained in Civilization One, we cannot accept that this state of affairs is a coincidence.

What we have with the Sumerian system is a fully integrated way of measuring length, volume, mass, area and time, using the same number bases in each case. The whole system can be constructed from a pendulum set by the movements of Venus across one degree of arc of the horizon or else from the bottom up with nothing m ore complicated than barley seeds.

The real question has to be whether or not the Sumerians them selves could have possibly known just how incredible their measuring systems were?

We are left with the impression that the system would have been very useful in the marketplace and on the farm in order to ensure equity of measurement throughout Sumerian society but that it is highly unlikely that the Sumerian Priests could have known the dimensions of the Earth, let alone its m ass. It is most likely that both concepts would have been absolutely alien to them.

This appears to be yet another example of direct and deliberate intervention into the development of humanity. In other words, as their own mythology demonstrates, someone 'taught' the Sumerians about weights and measures and told them the numbers to use.

By so doing they supplied the Sumerians with one of the hallmarks of true civilization, namely an integrated and replicable measuring system. At the same time, the use of the barley seeds added to a significant series of messages about these events in prehistory that were intended for our consumption.

Since it seems unlikely that a cereal grain as widespread and useful as barley could, by chance, behave in the way that it does in terms of its size and weight, it seem s very likely to us that the crop was genetically engineered.

It was used by the Sumerians for bread but also brewed into a beer that was drunk for m any centuries across the whole of civilization.
 

Back to Contents