Chapter Two

Hyperdimensional Physics

One of the first things Hoagland and Torun noticed was that throughout the observed solar system, planetary disturbances and upwellings of energy seemed to preferentially cluster around this key 19.5° latitude.

In addition to Neptune's Great Dark Spot (as it came to be known), the Great Red Spot of Jupiter, the erupting volcanoes of Jupiter's moon Io, Olympus Mons on Mars (the largest shield volcano in the solar system - below) and Earth's own Mauna Kea volcano in Hawaii all were at, or very near, 19.5° latitude [Fig. 2-1].

Beyond that, clusters of sunspots occurring from the heightened energy output of the sun during the peak of the solar cycle also centered around 19.5°.

And interestingly, whether the upwelling event was located in the north or south hemisphere depended on the alignment of the bodies' magnetic field. If the field were anchored at the South Pole, the disturbance appeared at or around 19.5° in the northern hemisphere.

Conversely, if it were anchored at the North Pole, the disturbance appeared in the south. It was as if there really were "giant tetrahedrons" inside the planets, driving the physics of these energy upwellings and forcing them to comply with some mysterious, unseen rules of behavior.



Excess Heat

Another key observation Hoagland and Torun made in these early days was the role that this theorized "tetrahedral physics" might play in another ongoing mystery of the outer solar system.

Beginning in the mid 1960s, ground-based telescopic observations of the solar system began to turn up the startling detection of anomalous internal infrared radiation coming from the planet Jupiter [Fig. 2-2].

Later Pioneer and Voyager spacecraft observations across the 1970s and '80s added the other "gas giant planets," Saturn, Uranus and Neptune, to the list of solar system worlds that somehow, without internal nuclear fusion processes (like stars) still managed to radiate more energy out into space than they receive directly from the sun (below).³⁰

After much initial debate, the conventional understanding of these anomalous "infrared excesses" eventually settled on three possible internal sources:

1. Primordial Heat. Leftover "fossilized thermal echoes" from the enormous energy associated with the accretion and collapse of the planet during its formation. Under this scenario, this energy was still stored inside the planet after literally billions of years, but slowly being radiated into space.

    

2. The Helium Drip Model. Heating caused by eventual internal separation of light elements in so-called "gas giant" planets (helium from hydrogen), releasing potential energy as the helium falls further toward the center of the planet (a form of ultra-slow, continued gravitational contraction).

    

3. Radioactive Decay. Anomalous energy release due to excess radioactive decay of heavy element concentrations located within massive gas giant "rocky cores."

Of the three conventional explanations for these "energy anomalies," only the first applies to Jupiter.

Because of its mass (318 "Earths"), Jupiter just barely falls into a category of worlds that could retain such primordial heating for the lifetime of the solar system (almost five billion years) and still be able to radiate observable heat.

But when scientists got around to actually measuring the amount of excess heat Jupiter radiates, it quickly became obvious that the "primordial heat model" was inadequate to account for Jupiter's infrared emissions. Even today, the current ratio of absorbed solar energy to emitted (five billion-year-old) internal Jovian energy is still almost two to one.

This is far in excess of what would be expected after such an enormous amount of time.

After the Voyager fly-bys of the 1980s, the second "internal heat" proposal - the "helium drip model" - was favored for the heat excess observed from Saturn. But, because of their relatively light masses (less than thirty times the Earth's), only the third possibility - massive internal radioactive decay - has been seriously attempted as an explanation for Uranus' and Neptune's more puzzling anomalous infrared emissions.

There are, however, serious problems with all of these conventional explanations for all planets less massive than Jupiter.

During the Voyager encounters of Uranus and Neptune, spacecraft instruments detected a barely measurable (but significant) "infrared excess" for Uranus of about 1.14 to 1. Whereas for Neptune, essentially Uranus' planetary "twin," the ratio of internal heat to intercepted sunlight was a striking three to one.

However, simultaneous Doppler tracking gravity measurements conducted during the fly-bys detected no anomalous central concentrations of heavy elements in either planet. This would be required if the excess observed IR radiation is, in fact, caused by excessive internal radioactive element concentrations.
 

Having failed to confirm the radioactive decay model, the conventional planetary physicists searched around for an alternative explanation for Uranus' excess energy output. They quickly seized on the one characteristic that separates Uranus from all other bodies in the solar system - its pronounced "axial tilt."

Compared to all the other planets of the solar system Uranus has an "obliquity" (the technical term) some 98° to the plane of its orbit of the sun; Neptune's is much more "normal": about 30°. (In comparison, Earth's obliquity is about 23.5°.)

This led to a new proposal, the "recent collision model."

This asserted that Uranus - somehow, long after its formation - suffered a massive impact with another major object, perhaps an errant moon. This, according to the theorists, in addition to accounting for the current "tipped over situation" of the planet, would have also added a significant amount of geologically "recent" internal energy to Uranus, driving up internal temperatures by equivalent amounts. This model argues that these resulting elevated temperatures in Uranus, derived from a massive cosmic collision, could thus account for Uranus' current infrared excess, as observed by Voyager in 1986.

Unfortunately, this explanation also quickly ran into difficulties. For one thing, Uranus is barely radiating "over unity" (more energy coming out than is going in) at its distance from the sun, while Neptune is radiating almost three times more energy "out" than it's getting from the sun.

When these two planets are "normalized" (i.e., when their differing distances from the sun are taken into account), their absolute internal "over unity" energy emissions are, in fact, just about the same. If the recent collision model was correct, Uranus should be radiating far more than Neptune. In fact, there is virtually no difference.

If a moon or other large object had recently struck Uranus, it certainly was not responsible for the planet's excess heat emissions.

What Hoagland then began to consider was that there might be an external source for this anomalous heat output, perhaps the same source that was fueling the 19.5° upwellings - but what could be the source of this mysterious excess energy that seemed to defy conventional explanation and conform to occult geometric rules?



Hidden History

At this point, Hoagland and Torun had a conundrum.

They had certainly developed a series of observations and connections that demanded closer scrutiny - but in what context? It was not enough to argue that the Cydonia ruins imparted knowledge of tetrahedral geometry and that this geometry seemed to have some reflected physical effects in the rotating bodies of the solar system.

They had to have a coherent model of the mechanism that was driving all of these observed planetary upwellings and the anomalous heat output. The pattern itself implied that there was an underlying physics involving the energy upwellings.

There is a perfectly natural explanation for such "anomalous energy" appearing in celestial bodies - unfortunately, it hasn't been seriously considered by science for over a hundred years. Hoagland found that the idea that "forces" - like gravity or magnetism - could be modeled geometrically was becoming quite popular within modern mathematics.

With that in mind, he began to look back at physics theories from the 1800s, and found that the very father of modern day physics, James Clerk Maxwell, had indeed dabbled in some equations that seemed to fit what he and Torun had observed on the outer planets.

Maxwell had routinely argued that the only way to solve certain problems in physics was to account for some phenomena as 3D "reflections" of objects existing in higher spatial dimensions. When Maxwell died, this higher dimensional or "scalar" component was stripped out of his equations by Oliver Heaviside, and the resulting "classic Maxwell equations" became the basis for our modern day models of electromagnetic force.

But if Maxwell's original work was correct - even though it had been discarded - then that meant his original concepts could potentially explain the various planetary phenomena Hoagland and Torun had observed. Tentatively, Hoagland began to examine more closely this early model of "hyperdimensional physics."

Hoagland found that a number of modern day mathematicians had actually begun to model these possible higher dimensions geometrically.

"Topologists" like H.S.M. Coxeter had done a significant amount of work in mapping the higher dimensional properties of a rotating "hypersphere," a sphere that exists in more than just three spatial dimensions.

The arcane mathematics describing this "hypersphere," and the multiple dimensions above it, are so complex that they are virtually unintelligible to all but the most educated math buffs. Yet the signatures of this higher dimensional physics - as reflected in our own 3D universe - are much easier to spot and predict.

His equations predicted that such a figure - if it was rotated - would create roiling vorticular motions (exactly like the observed dynamics of Jupiter's "Great Red Spot") in the sphere's 3D geometry, and at a specific latitude - 195°.

This was exactly what Hoagland and Torun were seeing in their own observations of the rotating planets and moons of the solar system. If these observations were indeed linked to the higher dimensional properties of the "rotating hypersphere," then it meant not only that rotating planets existed simultaneously in multiple, higher spatial dimensions, but also that this new physics could provide potentially limitless amounts of energy to drive the observed dynamics of their atmospheres, their internal fluid motions, their surface geological "upwellihgs" - everything! Even, ultimately, "life" itself...

The cornerstone of the hyperdimensional model is the notion that "higher" spatial dimensions not only exist, but are also the underlying foundation upon which our entire 3D reality exists.

Beyond that, everything in our observable 3D world is, in fact, driven by mathematically modeled "information transfer" from these higher dimensions. This "information transfer" might simply be the result of changes in the geometry of a connected system, say a change in the orbital parameters of a planet, like Jupiter or the Earth.

Since we are limited in our perceptions to the 3D universe we live in, we cannot "see" these higher dimensions.

However, we can see (and measure) changes in these higher dimensions that have simultaneous effect on our reality. By definition, this change in higher dimensional geometry is perceived in our 3D universe as an "energy output" - like the various 19.5° planetary energy upwellings we have noted previously.

The hyperdimensional model therefore implicitly argues that, contrary to Einstein's dictates, instantaneous "action at a distance" in our universe is indeed possible - in fact, it is a given - because of this higher dimensional information transfer. The model predicts that the effects of a "cause" in our three dimensions, whatever it may be, can be felt in measurable and predictable ways in our perceived universe at speeds incomparably greater than that of light.

The universe accomplishes this seemingly impossible feat by the transformation and transfer of information (as a different "energy") through "hyperspace," i.e. through these higher spatial dimensions. In our familiar three dimensions, this information/energy is then transformed back into familiar energy forms, like light, heat and even gravity itself.

Changes in one gravitationally-connected system on a large scale, like the planetary scale of a solar system, can therefore have an instantaneous, measurable effect on other bodies in the same system - providing there is a "resonance condition" (a "matched" connection) between those two objects via hyperspace.

Thus, the hyperdimensional model argues that everything, even widely separated three-space objects like remote planets, are ultimately connected through this four-space interaction; meaning that a "cause" in one place (like Jupiter) can have an "effect" in another (like the sun) - without any measurable 3D force (such as an electromagnetic wave) having measurably traversed the three-space distance in between.

Mainstream physics teaches us that this phenomenon - called "non-locality," which has been observed for decades in laboratory experiments ³² is simply a baffling "quantum reality" limited to ultra-short distances at the sub-atomic level, but that it does not, and cannot, affect larger objects at great distances (like planets, stars or galaxies themselves).

Because the speed of light is theorized to be the absolute speed limit of our 3D macro-universe, no cause is supposed to have a measurable effect on any object anywhere sooner than it takes light to travel the distance in between.

Yet these "magical," faster-than-light signals between atomic particles, and even communication between photons, has now been overwhelmingly confirmed at macro distances as well. Our current understanding of the limiting speed of light, based on Maxwell's equations of electromagnetism, asserts that only certain kinds of energy, like electromagnetic radiation, can even travel long distances through the vacuum of space.

In this classical "Einsteinian" view of physics, there is no "ether" - as it was called in Maxwell's day - to carry electromagnetic radiation's transverse waves across the vacuum.

In the hyperdimensional model, the ether is back-as the actual transformation medium between the higher spatial realities and our dimension - through something called the "torsion field" (The word "torsion" is formed from the same root as "torque" - and means "to spin").

So a torsion field is a "spin field" - a crucial point we will come back to.

The torsional-etheric field is, therefore, not the 19 -century's "electromagnetic ether" at all - but rather a spin-sensitive, geometric etheric state-whereby hyperdimensional information/energy is detectable in our dimension via spinning vorticular (rotating and/or precessing) physical systems.

Contrary to mainstream physics dogma, a rich series of experiments - for over a hundred years - have overwhelmingly confirmed various aspects of this non-electromagnetic "spin-field ether." The still developing mathematics and graphs that model this theoretical cosmology now, unfortunately, are as Byzantine and arcane as anything in science today.

However, this mathematics is reinforced by a veritable explosion of theoretical papers and fascinating laboratory experiments which have been secretly carried out in Russia for over 50 years - only becoming widely available here (via the Internet) with the collapse of the Soviet Empire.

So even though there is substantial and growing reason to suspect that the hyperdimensional/torsion model may ultimately be correct as the "Theory of Everything," most modern physicists (certainly in the West) still reject the notion, and stubbornly don't want to "go there."

Yet that was not always the prevailing sentiment in Western physics.

Hyperspace

The mathematical and physical parameters required for such "information/ energy gating" into this spatial dimension from potential "n-dimensions" were primarily founded in the work of several 19^th-century founders of modern mathematics and physics.

Among these were,

• German mathematician Georg Riemann

• Scottish physicist Sir William Thompson (who would eventually be knighted by the British crown as "Baron Kelvin of Largs" for his scientific and technological contributions)

• Scottish physicist James Clerk Maxwell

• British mathematician Sir William Rowan Hamilton

Georg Riemann mathematically initiated the 19^th-century scientific community (if not the rest of Victorian society) into the unsettling idea of "hyperspace" on June 10, 1854.

In a seminal presentation made at the University of Gottingen in Germany, Riemann put forth the first mathematical description of the possibility of "higher, unseen dimensions" under the deceptively simple title "On the Hypotheses Which Lie at the Foundation of Geometry."

Reimann's paper was a fundamental assault on the two-thousand-year-old assumptions of "Euclidian geometry" - the ordered, rectilinear laws of "ordinary" 3D reality. In its place, Reimann proposed a 4D reality (of which our 3D reality was merely a "subset"), in which the geometric rules were radically different, but also internally self-consistent.

Even more radically, Reimann proposed that the basic laws of nature in three-space, the three mysterious forces then known to physics - electrostatics, magnetism and gravity - were all fundamentally united in four-space, and merely "looked different" because of the resulting "crumpled geometry" of our 3D reality. In essence, he argued that gravity, magnetism and electricity were all the same thing - energies coming from higher dimensions.

Reimann was suggesting a major break with Newton's "force creates action-at-a-distance" theories.

These had been proposed to explain the "magical" properties of magnetic and electrical attraction and repulsion, gravitationally curved motions of planets and falling apples for over 200 years. In place of Newton, Reimann was proposing that such "apparent forces" are a direct result of objects moving through three-space geometry, distorted by the intruding geometry of four-space.

It is clear that Maxwell and other "giants" of 19th-century physics (Kelvin, for one), as well as an entire contemporary generation of mathematicians (like Cayle, Tait, etc.). took Reimann's ideas very much to heart; Maxwell's original selection of four-space "quaternions" as the mathematical operators for his force equations and descriptions of electrical and magnetic interaction, clearly demonstrate his belief in Reimann's approach - as does his surprising literary excursions into poetry, vividly extolling the implications of "higher-dimensional realities," including musings on their relationship to the ultimate origin of the human soul.

In 1867, following decades of inquiry into the fundamental properties of both matter and the space between, Thompson proposed a radical new explanation for the most fundamental properties of solid objects: the existence of "the vortex atom."

This was in direct contradiction to then-prevailing theories of matter, in which atoms were still viewed as infinitesimally,

"small, hard bodies [as] imagined by [the Roman poet] Lucretius, and endorsed by Newton..."

Thompson's "vortex atoms" were envisioned, instead, as tiny, self-sustaining "whirlpools" in the so-called "ether" - which Thompson and his contemporaries increasingly believed extended throughout the universe as an all-pervasive, incompressible fluid.

Even as Thompson published his revolutionary model for the atom, Maxwell, building on Thompson's earlier explorations of the underlying properties of this "etheric fluid," was well on the way to devising a highly successful "mechanical" vortex model of the "incompressible ether" itself, in which Thompson's vortex atom could live - a model derived in part from the laboratory-observed elastic and dynamical properties of solids.

Ultimately, in 1873, he would succeed in uniting a couple hundred years' worth of electrical and magnetic scientific observations into a comprehensive, overarching electromagnetic theory of light vibrations, carried across space by this,

"incompressible and highly stressed universal etheric fluid."

Maxwell's mathematical basis for his triumphant unification of these two great mystery forces of nineteenth century physics were "quaternions," a term invented (adopted would be a more precise description) in the 1840s by mathematician Sir William Rowan Hamilton, for,

"an ordered pair of complex numbers."

Complex numbers themselves, according to Hamilton, were nothing more than,

"pairs of real numbers which are added or multiplied according to certain formal rules."

In 1897, A.S. Hathaway formally extended Hamilton's ideas regarding quaternions as "sets of four real numbers" to the idea of four spatial dimensions, in a paper entitled "Quaternions as Numbers of Four-Dimensional Space."³⁴

According to Maxwell, action at a distance was possible through the "ether," which he defined as higher spatial dimensions - or what we now call "hyperspace." In other words, the father of modern terrestrial electromagnetic physics had come to the same conclusions as Hoagland's theorized "Martian architect" at Cydonia.

This may seem to be a tenuous connection at first, but when you read certain lines from his poem presented to the Committee of the Cayley Portrait Fund in 1887, it becomes quite clear, that he knew:

"Ye cubic surfaces! By threes and nines, draw round his camp your seven-and-twenty lines - the Seal of Solomon in three dimensions..."

This clear description of the "Seal of Solomon in three dimensions," is an overt reference to the geometrical and mathematical underpinnings of the infamous "circumscribed tetrahedral geometry" memorialized all over Cydonia.

If you take the base figure of a tetrahedron - the equilateral triangle - and add a second equilateral triangle to the figure exactly opposite the first, and then circumscribe that figure with a circle, you get the familiar "Star of David," the "Seal of Solomon" that Maxwell describes.

And in this figure, the tips of the double triangle touch the circle at the poles and at 19.5° north and south, directly linking the identical, hyperdimensional quaternion geometry whose physical effects we have now rediscovered all across the solar system.

And, of course, taking this "Seal of Solomon" and drawing it in three dimensions would give you a double-star tetrahedron circumscribed by a sphere [Fig. 2-4].

The reference to "seven and twenty lines" is also a not-so-subtle reference to a 2D sketch of a double tetrahedron encompassed by a "hypercube," shown as a base 2D form of a hexagon [Fig. 2-5].

The Heavy Hand of Heaviside

In a tragedy for science, two other 19^th-century "mathematical physicists," Oliver Heaviside and William Gibbs, "streamlined" Maxwell's original equations down to four simple (if woefully incomplete) expressions after Maxwell's death.

Because Heaviside openly felt the quaternions were "an abomination," - never fully understanding the linkage between the critical scalar (a directionless measurement, like speed) and vector (a directionally defined value, like displacement) components in Maxwell's use of them to describe the energy potentials of empty space ("apples and oranges," he termed them) - he eliminated over twenty quaternions from Maxwell's original theory in his attempted "simplification."

Oliver Heaviside was once described (by Scientific American) as,

"self-taught and... never connected with any university... [he] had [however] a remarkable and inexplicable ability to arrive at mathematical results of considerable complexity without going through any conscious process of proof."

According to other observers, Heaviside actually felt that Maxwell's use of quaternions and their description of the "potentials" of space was "mystical, and should be murdered from the theory."

By drastically editing Maxwell's original work after the latter's death, excising the scalar component of the quaternions and eliminating the hyperspatial characteristics of the directional (vector) component, he effectively accomplished this goal single-handedly.

This means, of course, that the four surviving classic "Maxwell equations" - which appear in every electrical and physics text the world over, as the underpinnings of all 20^th-century electrical and electromagnetic engineering - never appeared in any original Maxwell paper or treatise. And every invention, from radio to radar, from television to computer science to every "hard" science from physics to chemistry to astrophysics that deals with these electromagnetic radiative processes, are based on these supposed "Maxwell equations."

They are, in fact, Heaviside's equations, not Maxwell's.

The end result was that physics lost its promising theoretical beginnings as a truly "hyperdimensional" science over a century ago, and instead was saddled with a very limited subset of that potentially unifying theory, thanks to Heaviside.

The advocates of an ether-based model of force were dealt a greater blow in 1887, when the Michelson-Morley experiments effectively proved there was "no material ether." What was lost, however, because of Heaviside, was that Maxwell had never believed in a material ether himself - he was assuming a hyperspatial ether simultaneously connecting everything in the universe.

The major source of confusion surrounding Maxwell's actual theory, versus what Heaviside reduced it to, is its math - a notation system perhaps best described by H.J. Josephs:

"Hamilton's algebra of quaternions, unlike Heaviside's algebra of vectors, is not a mere abbreviated mode of expressing Cartesian analysis, but is an independent branch of mathematics with its own rules of operation and its own special theorems. A quaternion is, in fact, a generalized or hypercomplex number."

In 1897, Hathaway published a paper specifically identifying these hypercomplex numbers as "numbers in four-dimensional space."

Thus, modern physics' apparent ignorance of Maxwell's nineteenth century success - a mathematically based, 4D "field-theory" - would seem to originate from a basic lack of knowledge of the true nature of Hamilton's quaternion algebra itself.

And, unless you track down an original 1873 copy of Maxwell's "Treatise," there is no easy way to verify the existence of Maxwell's "hyperdimensional" quaternion notation; for, by 1892, the third edition incorporated a "correction" to Maxwell's original use of "scalar potentials," thus removing a crucial distinction between four-space "geometric potential," and a three-space "vector field" from all subsequent Maxwellian theory - which is why modern physicists, like Michiu Kaku, apparently don't realize that Maxwell's original equations were, in fact, the first geometric four-space field theory, expressed in specific four-space terms - the language of quaternions.

Rediscovery

One of the difficulties of proposing a "higher dimension" is that, inevitably, people (and scientists are people), will ask "well, where is it?!"

The most persistent objection to the four-space geometries of Reimann, Cay ley, Tait and Maxwell was that no experimental proof of a "fourth dimension" was readily apparent.

One of the more easily understandable aspects of "higher dimensionality" was that a being from a "lower dimension" (a 2D "Flatlander," for instance) entering our "higher" 3D reality, would appear to vanish instantly from the lower-dimensional world (and, consequently, appear just as suddenly in the higher dimension, albeit geometrically distorted.) When she returned to her own dimension, she would just as "magically" reappear.

To the scientific mind, however, people in our dimension don't just turn a corner one day and promptly vanish into Reimann's fourth dimension.

While mathematically derivable and beautifully consistent, to "experimentalists" (and all real science ultimately has to be based on verifiable, independently repeatable experiments) there seemed no testable, physical proof of hyperdimensional physics. Thus hyperspace - as a potential solution to unifying the major laws of physics - quietly disappeared, not to resurface until April 1919.

At that time, a remarkable letter was delivered to one Albert Einstein.

Written by Theodor Kaluza, an obscure mathematician at the University of Konigsberg in Germany, the letter's first few lines offered a startling solution (at least to Einstein, who was unknowledgeable of Maxwell's original quaternion equations) to one of physics' still most intractable problems, the mathematical unification of his own theory of gravity with Maxwell's theory of electromagnetic radiation via introduction of a fifth dimension.

(Because Einstein, in formulating the General and Special Theory of Relativity in the intervening years since Reimann, had already appropriated time as the fourth dimension, Kaluza was forced to specify his additional spatial dimension as a fifth. In fact, this was the same spatial dimension as the four-space designations used by Maxwell and his colleagues in their models over 50 years before.)

Despite its stunning mathematical success in apparently finally uniting gravity and light, the same question was asked of Kaluza as had been asked of Reimann over sixty years before. Because there was no overt experimental proof of the physical existence of another spatial dimension, Kaluza got the same "OK, where is it?" challenge to his assertions. Kaluza had a very clever answer: he proposed that the fourth dimension had somehow collapsed down to a tiny circle, "smaller than the smallest atom."

In 1926, another essentially unknown mathematician, Oskar Klein, was investigating the peculiar implications of Kaluza's ideas in the context of the newly invented atomic theory of quantum mechanics.

Klein was a specialist in the truly arcane field of mathematical topology - the higher dimensional surfaces of objects. Quantum mechanics had just been proposed a year or so before Klein's further topological investigation of Kaluza's ideas, by Max Planck and many others rebelling against perceived limitations of Maxwell's (now sanitized) electromagnetic theory.

The "quantum mechanics" theory would eventually become a highly successful (if bizarre, by common-sense standards) non-geometric effort to describe interactions between "fundamental particles," exchanging "forces" through discrete "quantitized" particles and energy in the sub-atomic world. Eventually, combining the two inquiries, Klein theorized that, if it truly existed, Kaluza's new dimension had likely collapsed down to the Planck length itself - supposedly the smallest possible size allowed by these fundamental interactions.

However, that size was only about "ten to the minus thirty-three" centimeters across.

Thus, the main obstacle to experimental verification of the Kaluza-Klein Theory (and the reason why people simply didn't "walk into the fourth dimension") was that quantum mechanics calculations affirmed that the only way to physically probe such an infinitesimally tiny dimension was with an atom smasher.

There was only one small technical problem:

The energy required would exceed the output of all the power plants on Earth, and then some.

Thus, the brief blip of new interest in hyperdimensional physics - the discussions of Kaluza-Klein among physicists and topologists - dropped through the floor by the 1930s.

This occurred both because of Klein's proof of the apparent impossibility of any direct experimental verification of additional dimensions, and because of the dramatic revolution then sweeping the increasingly technological world of big science.

There was at that time a flood of verifications gushing forth from atom smashers all around the world, feverishly engaged in probing quantum mechanics. The rapidly multiplying populations of "fundamental particles" spawned by this bizarre mathematical world led Einstein to refer to the theory as "sorcery."

Later, even though he accepted some of the experimental results, he remained skeptical that it was a complete answer to the questions posed by the physical universe.
 

Thirty more years would pass before scientific interest in hyperspace would be reborn as superstring theory - in which fundamental particles and "fields" are viewed as hyperspace vibrations of infinitesimal, multidimensional strings.

For most physicists currently interested in the problem, the superstring hyperdimensional model has overwhelming advantages over its predecessors. Besides effectively unifying all the known forces of the universe, from electromagnetism to the nuclear force, in a literally beautiful "ultimate" picture of reality, it also makes a specific prediction about the total number of n-dimensions that can form: ten or twenty-six, depending on the rotation of the "strings."

The bad news is that they can't be tested either, because all ten dimensions are curled up (in the model) inside the experimentally unreachable Planck length.

The hottest mainstream scientific theory to come along in more than half a century, the closest thing we have to a "Theory of Everything," is not only a hyperdimensional model of reality, it is yet another theory which, by its fundamental nature, can't be scientifically tested - while a hyperdimensional model which can be tested (and was, apparently, for decades behind the Iron Curtain) has been systematically ignored in the West for over a hundred years.



Tesla, Bearden and DePalma

As Hoagland continued to make new connections of the geometry of Cydonia with the historical treatment of hyperspatial realities, he encountered a number of independent, rouge experimentalists who had been working along these same lines.

Foremost among these were Dr. Bruce DePalma, an M.I.T. physicist and researcher, and Lt. CoL Thomas Bearden, a nuclear engineer and physicist who had been working on Maxwell's original model since his days on the U.S. Army's scalar weapons programs.

Bearden had tirelessly researched Maxwell's original writings, and concluded that Maxwell's original theory is, in fact, the Holy Grail of physics - the first successful unified field theory in the history of science.

Bearden had done dogged detective work to uncover Maxwell's papers, and from them had concluded that Heaviside had literally hijacked Maxwell's theory and set modern science back almost a hundred years. According to Bearden, not only would modern physics never find the single unifying element for gravity, electricity and magnetism (because it was all based on Heaviside's broken version of Maxwell's model), but that if the original model were restored, it had the potential to unleash nearly limitless amounts of energy, and to allow humanity the means to actually "engineer" forces like gravity at the quantum level.

This radical view was supported by Bearden's own research, which was based on papers and experiments carried out by Sir Edmund Whittaker and Nicola Tesla in the early 20th-century, and later confirmed in the so-called "Aharonov-Bohm" experiments.

Tesla, the inventor of modern civilization (through his discovery of alternating current), had conducted a number of relevant experiments in his lab in Colorado Springs in 1899.

During one experiment, he observed and recorded "interfering scalar waves." Via massive experimental radio transmitters he had built on a mountaintop in Colorado, he was broadcasting and receiving (by his own assertion) longitudinal stresses (as opposed to conventional EM "transverse waves") through the vacuum.

This he was accomplishing with his own, hand-engineered equipment (produced according to Maxwell's original, quaternion equations), when he detected an interference "return" from a passing line of thunderstorms.

Tesla termed the phenomenon a "standing columnar wave," and tracked it electromagnetically for hours as the cold front moved across the west. Tesla's experiments were suddenly stopped when his benefactor, J.P. Morgan, discovered the true purpose of his experiment - to generate unlimited amounts of electricity "too inexpensive to charge for."

Bearden was also interested in generating energy by creating "longitudinal stress" in the vacuum using Maxwell's quaternion/hyperdimensional potentials. Bearden wrote several papers on the theory, eventually published by the Department of Energy on their official website.³⁷

Bearden then set about the task of actually constructing a device that could draw "energy from the vacuum," eventually patenting a device (The "Motionless Electromagnetic Generator") that seems to generate energy from literally nothing.³⁸

Of course, you can't really get something from nothing, and Hoagland quickly realized that the effect Bearden was describing was the same "hyperdimensional" effect he was seeing in the heat generation of the outer planets.

There is now much fevered discussion among Western physicists on the Quantum Electrodynamics Zero Point Energy of space - "the energy of the vacuum."

To many familiar with the original works of Maxwell, Kelvin, et. al., this sounds an awful lot like the once-familiar "ether," merely updated and now passing under an assumed name. Described as some sort of exotic quantum effect to make it seem acceptable, this "zero-point energy" is nothing more than Maxwell's hyperdimensional physics in another guise.

Thus, creating and then relieving a "stress" in Maxwell's vorticular ether is precisely equivalent to tapping the energy of the vacuum that, according to current quantum mechanics models, possesses a staggering amount of such energy per cubic inch of empty space.

Even inefficiently releasing a tiny percentage of this "strain energy" into our three dimensions, or into a body existing in 3D space, could make it appear as if the energy was coming from nowhere - something from nothing. In other words, to an entire generation of students and astrophysicists woefully ignorant of Maxwell's original equations, such energy would appear as the dreaded "P" word... "perpetual motion."

As we shall show, it is this "new" source of energy - in a far more controlled context - that seems to also be responsible for not only the anomalous infrared excesses Hoagland has noted in the so-called giant outer planets of this solar system, but for the radiated energies of stars themselves.
 

Yet how does one create a "stress in the ether" to generate energy, or test this hyperdimensional physics theory? The theoretical notions of Maxwell and Bearden had already been tested, albeit inadvertently, by the aforementioned Dr. DePalma.

DePalma, brother of the famed film director Brian DePalma, long before he and Hoagland met, had been running (since the '70s) a series of groundbreaking "rotational experiments" which remarkably confirmed much of what Hoagland would be theoretically rediscovering twenty years later.

One practical invention was DePalms's "N-Machine" - a high-speed, "homo-polar generator" which is able to pull measurable electrical power literally out of thin air" (the vacuum) with no expenditure of fuel...

Among DePalma's many other radical results was an experiment in which he simultaneously ejected two metal balls - one spinning at over 27,000 rpm and one not spinning at all - from the same test rig (below).

He then measured the rate at which they both rose and fell. In shocking contrast to the expected result if standard "Newtonian" mechanics are at work, the spinning ball rose farther and faster, and fell to the ground faster, than the non-spinning ball - even though exactly the same upward force (momentum) had been applied to both [Fig. 2-5].

The implication was that the spinning ball had somehow managed to gain energy from somewhere - which simultaneously altered both the effects of gravity and inertia on it... exactly as Bearden's model had independently proposed.

DePalma conducted countless additional rotational experiments, including with massive gyroscopes, throughout the 1970s. In the course of these mechanics, he discovered that gyros, when spun up - and simultaneously-induced to mechanically precess (wobble on their axis of rotation), could also be used to substantially negate the effects of gravity. In one experiment, a 276-pound "force machine" was reduced in weight by six pounds - about a 2% loss - when the gyros were switched on.

Depalma also discovered that these massive rotating systems, even when carefully isolated, could induce "anomalous rotational motions" in other gyroscopic set ups, even in other rooms... but only if they were also rotating.

As a result of these years of painstaking laboratory experimentation, with a great variety of different spinning systems, DePalma ultimately argued that all rotating objects - including planets and stars - must intrinsically precess.

"Precession" is the tendency of spinning objects, like a child's top or a planet like the Earth, to wobble on their rotational axes. In conventional mechanics, precessional motion is explained as coming from an outside force (like the Moon's gravity tidally tugging on the Earth's slightly bulging equator) unbalancing an object's spin.

Based on DePalma's empirically-derived measurements of rotation, he predicted that even apparently isolated spinning objects would precess - by virtue of their interaction with other rotating objects.

They drew energy from some kind of non-magnetic, non-gravitational field (termed "the OD field"...), which he proposed had to exist to explain his perplexing "energy accreting" spinning ball experiment. Ironically, totally unknown to DePalma - because of the Cold War and the KGB's strict secrecy controls - what he was observing was also being simultaneously observed by his Russian counterparts, and termed "a torsion field" - based on the same rotational interactions.

The idea of "isolated precession" - the logical conclusion of DePalma's decades of observations of all kinds of anomalous spinning systems - has never been experimentally examined under controlled laboratory conditions (at least, in any published literature in the West...), as DePalma needed a "zero gravity" (force-free) environment to conduct the proper test.

Unfortunately, before Hoagland (with his NASA associations and connections) could conclude arrangements for just such test of Bruce's remarkable hypothesis, to be conducted at NASA-Lewis's vacuum zero-gravity drop test facility, DePalma suddenly died in 1998.

Ultimately, Depalma's fascinating prediction concerning intrinsic rotational precession - which can now be elegantly explained by invoking multiple rotational motions and torsion interactions occurring simultaneously in higher dimensions - will come to have great significance in our story later on.

With Bearden and DePalma's experimental input in hand, Hoagland began to consider serious methods of doing the one thing that all the other hyperdimensional theories had been unable to accomplish: carry out actual testing of their core assumptions.



A Testable Theory

The true scientific method is something that is woefully misunderstood in our modern world, even by many scientists today.

The history of science is replete with debates that have raged back and forth in colossal wars of ego and self-interest - but the method itself is supposed to protect us from scientists becoming a new priesthood, by making sure that when models don't fit the new data, they are discarded, no matter how appealing they may be to some interests.

Unfortunately, it rarely works that way.

Hoagland wanted to immediately separate his concept of hyperdimensional physics from the earlier models in one distinct way - prediction. Only if his new ideas could be either confirmed or falsified would his modern version of Maxwell's revolutionary ideas ever gain traction - and in order to accomplish that, the first order of business for any valid scientific model is to produce testable predictions based on that model.

Fortunately, there were several implicit tests of the hyperdimensional model almost immediately suggested by the original observations themselves. Eventually, Hoagland settled on four additional key predictions that would determine if Cydonia's "embedded tetrahedral physics" and the resulting "hyperdimensional model" would be falsifiable.

All of these tests would invariably spring from the same, somewhat surprising source.



Angular Momentum

Hoagland's first focus was on the anomalous heat emissions he and Torun had noted in the outer planets.

Since in three dimensions all energy eventually "degrades" to random motions, via Kelvin and Gibb's laws of thermodynamics, then "stress energy" of the ether (vacuum) released inside a material object, even if it initially appears in a coherent form, will eventually degrade to simple, random heat, ultimately radiated away as excess infrared emissions into space.

In the end, all energy, whatever its source, ends up looking the same.

Because of this, Hoagland focused on the initial, astrophysical conditions under which such "Maxwellian space potentials" can be released inside a planet or star. The idea was to predict a specific set of signatures that would uniquely identify the source of these energy emissions as hyperdimensional, versus a "normal" 3D effect.

In attempting to understand the anomalous IR radiation, one thing quickly became clear; to a first order, the infrared excesses of the giant planets all seemed to correlate very nicely with one parameter each has in common-their total system "angular momentum."

The mass of a body and the rate at which it spins, in classical physics, determines an object's angular momentum. In the hyperdimensional model, it's a bit more complicated, because objects apparently separated by distance in this dimension are in fact connected in a "higher" (four-space) dimension.
 

So, in the hyperdimensional model, one also adds in the orbital momentum of an object's gravitationally tethered satellites - moons in the case of planets, planets in the case of the sun or companion stars in the case of binary star systems.

In this view, then, the one advocated by Hoagland and derived from his "meaningless" observations of the mathematics of Cydonia, this total system angular momentum was the key to understanding how things really worked in our 3D universe. This is in stark contrast to the currently accepted view of field theory and electromagnetic force, which views a planet or star's mass as the most important characteristic dictating astrophysical behavior.

Because mainstream physicists are working with Heaviside's version of Maxwell's original concepts, the most significant "force" they can observe is gravity. Since gravity is driven by mass, modern physics has always assumed that mass is the single most influential aspect of astrophysical interactions.

However, when we measure total solar system angular momentum, we get something of a surprise... [Fig. 2-6]

Jupiter as it turns out, which has less than 1% of the mass in the solar system, somehow possesses 60% of the angular momentum, whereas the sun, which possesses 99% of the mass, has only 1% of the angular momentum. If the conventional view of the solar system is correct, then in fact the distribution of angular momentum to mass should be roughly equivalent.

Instead, it is completely flip-flopped.

Various notions have been floated to explain this theoretical discrepancy, including the idea that the sun somehow magically "transferred" its angular momentum to the planets - but there are big problems with all of these ideas, and formation theoreticians are not close to providing an answer.

Hoagland had first begun to examine the role angular momentum might play in his emerging theory when he made one significant association - the common link connecting all the objects for which the Cydonia "embedded tetrahedral model" works, from the planets to the sun, seemed to be based on a relationship between angular momentum and magnetic fields.

Before the adoption of the present, complex "self-excited dynamo theory" (with internal, circulating, conducting "fluids" as the mechanism for general planetary and stellar magnetism), another, strictly empirical, hypothesis was proposed - a strikingly simple relationship between the observed total angular momentum of the object, and a resulting magnetic dipole.

Termed "Schuster's Hypothesis" (after Sir Arthur Schuster [1851-1934], who made the original correlation and whose very name and empirical discovery is, inexplicably, completely missing from all NASA literature on planetary magnetism), it has been successful in predicting magnetic field strengths (Blackett 1947, Warwick 1971) ranging from the Earth's, to the sun's, to Jupiter's vast field (20,000 times the terrestrial dipole moment).

Schuster's prediction, made over sixty years prior to the 1973-74 Pioneer 10 and 11 close-up confirmations (Warwick 1976), led Warwick to comment thusly on the remarkably predictive power of "Schuster's hypothesis" in 1971:

"Dynamo theory has not yet successfully predicted any cosmical fields. Its use today rests on the assumption that no alternative theory corresponds more closely to observations."

Indeed, after Mariner 10 detected a magnetic field around Mercury that not only fit Schuster's hypothesis, but also directly contradicted the dynamo theory, even Carl Sagan admitted that there was a need for serious revision of science's view of planetary magnetism.

Taking Schuster's 1912 proposal, Hoagland and Torun plotted contemporary parameters for angular momentum versus the observed magnetic dipole moment (for all planetary objects now visited by spacecraft with magnetometers), and found Schuster's hypothesis confirmed - with the exception of Mars (which had its magnetic field stripped away in a recent catastrophe, which we will deal with later) and Uranus (discussed below).

The truth is, the dynamo theory has never produced a single correct prediction of any planetary magnetic field, and Schuster's Theorem has been correct in almost every case.

Uranus, as the sole exception to the accuracy of Schuster's Theorem, may in fact be the exception that proves the rule. Uranus has a rotational period almost exactly the same as Neptune's, and should by definition have a similar magnetic field strength.

However, Neptune has a magnetic field strength about half of Earth's while Uranus' is almost two thirds that of Earth's.

If Schuster's Theorem is correct, then the two planets' magnetospheres should be about the same intensity. Instead, there is an almost two-to-one ratio. Uranus, however, is exceptional in many other ways - its obliquity is almost 90° from solar vertical, implying that it may have suffered a significant pole shift in the recent past that could account for this discrepancy.

If this happened in the geologically recent past, then there would logically follow a period of non-compliance with the theorem.

Given that there was also a significant hyperdimensional factor (related to DePalma's tests with spherical precession) that may have accounted for Uranus' present condition, and since Schuster's observation works quite well for the other planets, it seems likely that there was an external factor not yet fully understood for Uranus' noncompliance - but clearly, if Schuster's Theorem is valid in seven out of nine cases and the Dynamo theory is zero for nine, Schuster's observation would appear to be a far closer fit.

The fascinating, observed correlation between angular momentum and magnetic dipole moment led Hoagland to make a similarly simple empirical connection to his own work.

When he looked at the relationship between the anomalous infrared emissions and angular momentum, he discovered that it too correlated precisely with each planet's total system angular momentum.

When one graphs the total angular momentum of a set of objects, such as the radiating outer planets of this solar system (plus Earth and the sun) against the total amount of internal energy each object radiates to space, the results are striking [Fig. 2-7].

The more total system angular momentum a planet (or any celestial body) possesses, the more anomalous energy it is capable of generating (actually, the energy - like the "anomalous energy" DePalma observed in his spinning ball experiment - is being "ducted" into the interior of the rotating mass from a higher dimension, via the 3D "etheric/torsion field").

For the "hyperdimensional physics" model, this simple but powerful relationship seems to be the equivalent of E=MC : a celestial object's total internal luminosity seems dependent upon only one physical parameter: luminosity equals total system angular momentum (object, plus all satellites).

What this implies is that the amount of energy a given object radiates is dictated by the force exerted on it through hyperspace, and that this hyperspatial energy is measurable in our 3D world as angular momentum. In graphing this fairly obvious correlation [Fig. 2-7], everything seemed to line up quite nicely. All the planets fell right where they should along the graph - the only exception was the sun. Somehow, the sun seemed to be missing a substantial quantity of its angular momentum.

The conventional view of the sun, and all stars like it, is that they are massive nuclear furnaces, fueled by the collapse of matter into a raging fireball of energy.

This process causes a literal fusion of atoms in the sun's interior, and should by implication produce fusion byproducts.

One such expected fusion byproduct is neutrinos, sub-atomic particles that lack an electrical charge. However, experiments to measure the sun's neutrino output have discovered that the sun is not emitting anything like the number of neutrinos required by the standard solar model for its observed energy emission. If its energy is due to "thermo-nuclear reactions" (as the standard model demands), then the observed "neutrino deficit" is upwards of 60%.

Even more remarkable, certain kinds of primary neutrinos (calculated as required to explain the bulk of the solar interior's fusion reactions, based on laboratory measurements) turn out to be simply missing altogether.
 

Recently, a combination of theoretical readjustments to existing quantum theory, coupled with the results of new neutrino detectors brought on-line, seem to have reconciled the observed "neutrino deficit" for the sun-bringing the observed number (and "flavor") back in line with the adjusted theory. But, there is the nagging suspicion on our part that this tinkering with the original neutrino/standard solar model - remember, created before the anomalous solar neutrino deficit had been observationally discovered - is a kind of "academic cheating"...

The answer to the sun's apparent violation of the standard solar model, ironically, is contained in its striking "violation" of our key angular momentum/ luminosity diagram. In the hyperdimensional model, the sun's primary energy source - like the planets' - must be driven by its total angular momentum, its own "spin momentum" plus the total angular momentum of the planetary masses orbiting around it.

As we pointed out above, though the sun contains more than 98% of the mass of the solar system, it contains less than 2% of its total angular momentum.

The rest is in the planets. Thus, in adding up their total contribution to the sun's angular momentum budget, if the hyperdimensional model is correct, we should see the sun following the same line on the graph that the planets, from Earth to Neptune, do. It does not.

The obvious answer to this dilemma is that the hyperdimensional model is simply wrong. The less obvious conclusion is that we're missing something-like additional planets.

In trying to account for the missing angular momentum, Hoagland had found his first testable prediction of the hyperdimensional model. By adding another big planet (or a couple of smaller ones) beyond Pluto (several hundred times the Earth's distance from the sun), the sun's total angular momentum could move to the right on the graph, until it almost intersects the line (allowing for a percentage, about 30%, of internal energy expected from genuine thermonuclear reactions).

This creates the specific prediction that the current textbook tally of the sun's angular momentum is deficient for one seemingly obvious reason: we haven't found all of the major planets in the solar system yet.

So the first implicit prediction of the hyperdimensional model was that eventually, observatories would find one massive planet in a prograde orbit, or two smaller solar system members in retrograde orbits.

Either observation, within certain limits, would allow the sun to move to its predicted location on the graph and confirm the correlation between energy output and angular momentum.

Yet there was one even bigger implication of the relationship between angular momentum and energy output. If the connection was real, then it means that our view of the hierarchy of the solar system is completely backwards. In the hyperdimensional model, the tail (the planets and moons) wags the dog (the sun)... an assumption that would begin to have far-ranging implications.



Confirmation?

The next step in testing this aspect of the model was to begin a search for any evidence that another member of our solar system might conceivably exist.

For years, there has been an ongoing search for "Planet X" in astronomy. This search has grown out of the observation that something, presumably a large undiscovered planet, seems to exhibit an influence on the orbits of Neptune and Uranus. The search for this "perturber" eventually led to the discovery of Pluto, but no such large planet has yet been found, at least not officially.

There have, however, been some very interesting "unofficial" discoveries that may have a bearing on this prediction. In 1982, a front-page article appeared in the Washington Post,40 including an interview with JPL's Dr. Gerry Neugebauer about an object spotted in Orion by the IRAS infrared satellite, at an estimated 50 billion miles from Earth.

This object fit Hoagland's prediction within very tight parameters.

To date, no follow-up observations or papers have been published on this object, and in inquiries to Dr. Neugebauer, he stated that the quotes in the story were "taken out of context. I do not know about it nor any follow-up."

Neugebauer strained credulity with this reply. How many of us would claim they didn't know anything about a subject for which we had been featured in a Washington Post article? When you read the actual interview and story, which is clearly based on Neugebauer and Dr. James Houck's information, it seems clear that they are not coming clean. The article describes the object as small and dark, about the size of Jupiter, and at a range of 50 billion miles from Earth.

At this distance (about 537 A.U. Astronomical Units), the object would probably have to be a brown dwarf, a body roughly the size of Jupiter but 50 times as massive. The Post article goes on to say that "two different telescopes" were enlisted to do a visual search for the object - yet Neugebauer insists it was never followed up.

Clearly, there seems to a blanket of denial that has now fallen around this early IRAS observation.

In 1999, evidence for yet another solar system member, this time in Sagittarius (exactly opposite Orion in the celestial sphere) was cited in several news reports.41 In this case, the object was assumed to be about the same size as the IRAS object, but many times more distant - between 25,000 and 32,000 A.U. Its presence was implied by the patterns of long-period comets.

Both of these observations show that Hoagland's prediction of additional planet(s) found at great distances from the Earth is at least somewhat supported by various observations. What separates Hoagland's model from all the other Planet X theories is the specific prediction that the object in question - when it is finally officially acknowledged - will possess enough angular momentum to move the sun to its proper place on the graph.

But what has to be questioned is if NASA does find our missing tenth major planet - Pluto's recent demotion to mere "solar system object" notwithstanding - will they tell us? There are numerous rogue researchers who have long predicted the existence of Planet X, and to admit that it had been discovered would give credence to their models and theories.

As we dug in deeper on this mystery, it became obvious that NASA's reasons for quieting Neugebauer had far more to do with politics (as usual...) than science.

Infrared Variability

The next implicit test of the hyperdimensional model also grew out of the infrared emissions observation.

If Hoagland and Schuster's observations were right, and there was a direct connection between luminosity, magnetic field intensity and angular momentum, then this had some fairly stunning implications.

Since the infrared emissions in Hoagland's model were implicitly presumed to be hyperdimensional in nature - i.e. connected to higher spatial geometries - then orbital changes in the configuration of the "system" (the constantly moving planets and moons of the solar system) should by implication lead to varying outputs of energy - like turning a rheostat up and down to control the output of a light.

This is a crucial point, since conventional physics, by rote, demands that energy outputs for these planets be "constant," perceptibly decaying only in the very long term.

If the ultimate source of planetary (or stellar) energy is this vorticular (rotating) spatial stress between dimensions (a la Maxwell), then the constantly changing pattern (both gravitationally and dimensionally) of interacting satellites in orbit around a major planet/star, coupled with its equally changing geometric configuration vis-a-vis the other major planets of the system, must modulate that stress pattern as a constantly changing, geometrically twisted "ether."

In Hoagland's hyperdimensional model, it is this constantly changing hyperspatial geometry that is capable of extracting energy from this underlying rotating, vorticular ether and then releasing it inside material, rotating objects.

Initially, this excess energy can appear in many different forms - highspeed winds, unusual electrical activity, even enhanced nuclear reactions - but, ultimately, it must all degrade to simple excess heat.

Because of the basic physical requirement for resonance in effectively coupling a planet (or a star's) rotating 3D mass to the underlying 4D ether rotation, this excess energy generation must vary with time, as the changing orbital geometry of the "satellites" and other major members of the solar system interacts with the spinning primary (and the underlying vorticular ether) in and out of phase.

For these reasons, time variability of this continuing energy exchange must be a central hallmark of this entire hyperdimensional process. It should also be straightforward to determine.

All that is required is to take measurements of Jupiter's IR output at various points in time along its orbit and at different positions relative to the other planets. If the hyperdimensional model is correct, then differing orbital configurations should make Jupiter's (and all other "gas giant") IR output(s) highly variable.

At certain times, it should be more than its canonic two to one output. At others, it should be less [Fig. 2-8].

Again, the historical scientific record provided considerable support for this.

Dr. Frank J. Low made the first observations of Jupiter's anomalous heat output from highflying aircraft in 1966 and 1969. Low, considered the father of modern infrared astronomy, published the early results that showed Jupiter's output was in the 3-1 range.⁴² He later went on to create the proposal that led to the creation of IRAS, the first infrared space telescope that made the IR observations of the possible "Planet X" in Orion that we discussed earlier.

Three years later, Low made further observations that reduced the figure from 3-1 to 2-1, a better than 30% difference that was far beyond the margin of error of the instruments in use on both occasions.

Later ground based telescopic observations during the 1970s revised the figure even further downward, to about a 1.67-1.00 ratio, a further 30% reduction! ⁴³ The Voyager missions largely confirmed the 1.67 ratio in the early 1980s. The assumption, despite the wide discrepancy, was simply that the earlier instruments were crude and their measurements were just way off.

Since the energy output had seemed to stabilize around the 1.67 figure in the late 1970s and early '80s, it was generally assumed this was the case and that the earlier results were written off.

Fortunately, there have been a couple of probes to the outer solar system since the Voyager days, Galileo and Cassini, and both carried instruments that could measure the infrared emissions of the outer gas giant planets. So the only thing keeping Hoagland from testing this aspect of the Model is in getting someone to take the measurements, or to release them if they did take them.

That has proven to be a harder task than one might imagine.

Calls to the universities where the infrared data for both spacecraft are gathered and archived resulted in a distinct lack of co-operation. Hoagland was told that in order to get the figures for the measurements, he would have to "prove" his affiliation with an "approved" research center or university. However, a search of NASA's online astrophysics abstract database yielded some interesting information.

In a recent paper, observations made with Cassini's Composite Infrared Spectrometer (CIRS) seemed to offer confirmation of Hoagland's prediction.44

The team of researchers found that Jupiter's infrared emissions did not fit the expected "1.67 to 1.00" ratio that had been the canonic number since Voyager.

While it does not give exact numbers, the abstract states that,

"the equatorial temperature minimum is much more pronounced than observed at the time of Voyager," and that "it is likely related to the temporal variation in the equatorial stratospheric temperatures that have been reported from ground-based observations."

Not only does Jupiter appear to be continuing to display variable heat output consistent with Hoagland's model, but the latter statement also implies that more recent ground based observations have been seeing the same thing.

While we won't go so far as to claim a cover-up of the more recent thermal imaging data for Jupiter and the other outer planets, clearly the results are anomalous for the conventional models and consistent with Hoagland's key HD prediction.

Unfortunately, we will have to wait for actual published numbers before we can rack this prediction firmly in the "confirmed" category.



Short-Term Amplitude Variations

This same aspect of the model could also be applied on a smaller scale to produce yet another prediction. In our own planetary system, all the "giant" planets possess a retinue of at least a dozen satellites: one or two major ones (approximating the size of the planet Mercury), with several others ranging down below the diameter and mass of our own moon, in addition to a host of smaller objects.

Because of the "lever effect" in the angular momentum calculations, even a small satellite orbiting far away (or at a steep angle to the planet's plane of rotation) can exert a disproportional effect on the "total angular momentum" equation-just look at Pluto and the sun [Fig. 2-6].

Even now, Jupiter's four major satellites (which have collective masses approximately 1/10,000th of Jupiter itself), during the course of their complex orbital interactions, are historically known to cause time-altered behavior in a variety of well-known Jovian phenomena - including "anomalous" latitude and longitude motions of the Great Red Spot itself.

As Hoagland presented at the U.N. in 1992, the Great Red Spot (GRS) - a mysterious vortex located for over 300 years at that infamous 19.5° S. Latitude, via the circumscribed tetrahedral geometry of the equally infamous twenty-seven line problem is the classic "signature" of hyperdimensional physics operating within Jupiter.

The existence of decades of recorded anomalous motions of this Spot, neatly synchronized with the highly predictable motions of Jupiter's own "Galilean" moons are clearly not the result of conventional gravitational or tidal interactions, given the relatively insignificant masses of the moons compared to Jupiter itself.

Rather, they seem to be following the models of Maxwell, Schuster and Whittaker; the result of powerful hyperdimensional modulation from the changing geometric configurations of these same satellites.

It is the long lever of angular momentum and harmonic torsion resonance on the constantly changing, vorticular scalar stress potentials (torsion field states) inside Jupiter that are causing the changes in the GRS.

So, hyperdimensional test number three: look for small, short-term amplitude variations in the infrared emission levels of all the giant planets, synchronized (as are the still-mysterious, but cyclic atmospheric motions of the GRS on Jupiter) with the orbital motions and conjunctions of their moons, and/or, the motions of these outer planets relative to the other major members of the solar system.

Confirmed short-term variations in the current planetary IR outputs of a few hours (or even a few days') duration - and synchronized with the orbital periods of the planets' satellites themselves - would thus be stunning evidence that all the mainstream explanations are in trouble, and that the hyperdimensional model deserves much closer scrutiny.

Rising or falling output over years and decades (as strongly implied by the historical IR observations of Jupiter, from Frank Low to Cassini) would support a longer-term, planetary modulation of these internal HD energy releases.

In fact, of course, both sets of modulations should be occurring simultaneously - easily separated via a suitably written computer observation program ... providing someone even looks.

These changing interactive stresses in the boundary between hyperspace and "real" space (in the hyperdimensional model) now also seem to be the answer to the mysterious "storms" that, from time to time, have suddenly appeared and disappeared in the atmospheres of several of the outer planets.

The shrinkage and virtual disappearance in the late 1980s of Jupiter's Great Red Spot is one remarkable example; as was Saturn's abrupt production of a major planetary "event," photographed by the Hubble Space Telescope in 1994, as a brilliant cloud erupting at 19.5° N (where else?); Neptune's "now you see it now you don't" major "19.5 storm" - the Great Dark Spot - is yet another.

And, in the latest solar system mystery to confound NASA theorists, there is the sudden formation of a second red spot, nicknamed "Junior" which occurred on Jupiter in 2006. This massive (Earth-sized), atmospheric vortex coalesced over a few weeks from three smaller vortices (each about the size of Mars) and then immediately began also turning "GRS red."

And NASA is admittedly "clueless" (again) to what is actually going on ....

Since the prevailing NASA view is that these planets' excess IR output must be constant over time, no one has bothered to look for any further correlations between a rising or falling internal energy emission and the (now historically well-documented) semi-periodic eruptions of such "storms" - and they should.
 

This whole notion, that the changing configuration of a planet's (or star's) system members relative to the "primary," can have an effect on its total energy output, is revolutionary to current thinking, but hardly without precedent.

There is a very well known, long period and still mysterious variability associated with the largest "hyperdimensional gate" in our own neighborhood - the sun.
Its complex changes, which include a host of related surface phenomena-solar flares, coronal disturbances, mass ejections, etc. - is termed "the sunspot cycle," because the number of simultaneous "spots" (lower-temperature vortices appearing dark against the hotter solar surface, as this activity occurs) waxes and wanes over about 11 years.

The full magnetic reversal of the sun's polarity takes two complete sunspot cycles to return to "zero" - thus the complete "solar cycle" is somewhat over twenty years.

In the 1940s, the Radio Corporation of America (RCA) hired John Nelson, a young electrical engineer, in an effort to improve the reliability of short-wave radio communications around Earth. Such radio transmissions had been observed to be more reliable in the "lulls" in between solar activity associated with "peak" sunspot years.

To his surprise, Nelson soon specifically correlated this rising and falling radio interference with not only the sunspot cycle, but with the motions of the major planets of the solar system. He found, to his increasing astonishment, a very repeatable - in essence, astrological - correlation between the inexorable orbits of all the planets (but especially, Jupiter, Saturn, Uranus and Neptune, which hold essentially all the solar system's known angular momentum) and major radio-disturbing eruptions on the sun.45

The hyperdimensional model finally provides a comprehensive theoretical explanation - a "linking mechanism" - for these (to a lot of astronomers) still embarrassing decades-old RCA observations.

For, in essence, what John Nelson had rediscovered was nothing short of a "hyperdimensional astrology" - the ultimate, very ancient, now highly demonstrable angular momentum foundations behind the real influences of the sun and planets on our lives.

Nelson also "rediscovered" something else:

"It is worthy of note that in 1948, when Jupiter and Saturn were spaced by 120°, and solar activity was at a maximum, radio signals averaged of far higher quality for the year than in 1951 with Jupiter and Saturn at 180° and a considerable decline in solar activity.

 

In other words, the average quality curve of radio signals followed the cycle curve between Jupiter and Saturn rather than the sunspot curve..."

These decades-old observations are very telling... not only confirming Jupiter and Saturn as the primary "drivers" behind the sun's known cycle of activity (in the hyperdimensional model), but strongly implying an additional direct effect of their changing angular relationship on the electrical properties of Earth's ionosphere.

This, of course, is totally consistent with these changing planetary geometries affecting not just the sun, but the other planets simultaneously as well, just as "conventional" astrologers have claimed, via Maxwell's "changing scalar potentials."

At this point, then, only the hyperdimensional theory:

1. Points to the deepest implications of the simple astronomical fact that the "tail wags the dog" - that the planets in this physics are fully capable of exerting a determinant influence on the sun, and each other, through their disproportionate ratio of total solar system angular momentum: over 100 to 1, in the (known) planets' favor.
    

2. Possesses the precise physical mechanism - via Maxwell's "changing quaternion scalar potentials" - accounting for this anomalous planetary angular momentum influence.
    

3. Has already publicly identified, at the United Nations in 1992, a blatant geometric clue to this entire hyperdimensional solar process: the maximum sunspot numbers (those large, relatively "cool," rotating vortices appearing on the solar surface), rising, falling and methodically changing latitude, during the course of the familiar twenty-two-year solar cycle - and peaking every half-cycle (around eleven years), at the solar latitude of about 19.5°.

Pulsars

Pulsars are yet another area where the hyperdimensional physics theory can be put to the test.

Hoagland and Torun predicted that pulsars, because of their incredible angular momentum and magnetic properties, should be excellent hyperdimensional physics test beds. In fact, in the case of one specific pulsar, they may be a major key in validating the overall Hoagland/Torun model [Fig. 2-9].

B1757-24, a pulsar first observed in July 2000, was found to possess far more angular momentum than it should have. In fact, the object defies all known and accepted "laws of physics" and seems to be tapping additional angular momentum from an unseen source.

That unseen source, according to Hoagland and Torun's prediction, is higher dimensional energy released by the rapid rotation of the pulsar.

Under the conventional physics model, stars are assumed to be "born" from spinning gas and dust nebulae. As they contract (under gravity), like an ice skater tucking in her arms, they must spin faster. This is the central tenet of a fundamental law of (current) physics, called "the conservation of angular momentum."

The only way a star is supposed to be able to get rid of this fixed quantity of angular momentum transferred to it at birth, is to "re-transfer" the momentum to space through one of basically two means: direct mass loss and/or magnetic interactions (accelerations) between the star and any surrounding nebulae or bodies (such as a companion set of planets or another orbiting star).

For most of a star's "main sequence" life, the period when it is assumed to be relatively stable in its spin and energy output (although the hyperdimensional model states that this output also is not "constant" or completely "stable" - but that's another argument), these mechanisms are supposed to be able to transfer at best a few percent of the star's original angular momentum.

So, a star at the end of its billions-of-years-old life is supposed to have pretty much the same quantity of angular momentum as it was originally born with.

When a massive star (between five and twenty times the mass of the sun) reaches the end of its life (defined in the conventional models as "the exhaustion of its nuclear fuel"), it goes supernova. In these models, roughly ninety percent of the outer parts of the star leaves by this means (ultra-rapid mass transfer into space - in excess of 5,000 miles per second!), leaving the remaining, collapsed, ultra-dense core behind, as a now rapidly-spinning "neutron star."

Such spinning, incredibly dense objects (essentially, the mass of the sun and the density of an atomic nucleus, smashed into a volume about the width of a small city) are supposed to be at the heart of the "pulsar phenomenon."

Thus, when "born" in this violent end-process of stellar evolution, such a rapidly spinning object is supposed to have been given (through the previous mechanisms) a finite quantity of angular momentum - not as much as the original star (because of the large fraction of mass lost in the explosion, taking that angular momentum with it), but just as finite.

In the ensuing "pulsar phenomenon," such a spinning, highly magnetized object is far more likely to interact with other nearby gas clouds, etc., than the original star. This is because the original magnetic field of the whole star is also supposed to be conserved, and is now collapsed down to the new volume of a "city-sized" object, from an original volume perhaps several trillion times as large.

Such incredibly high-strength magnetic fields are then supposed to be able to accelerate matter still in the vicinity of this newly-born, rapidly spinning object (the outwardly exploding shell of the original star) and fling some of it away from the star via "magnetic acceleration" at an appreciable fraction of the speed of light.

This phenomenon is what's supposed to create the accelerating beams of matter that spin with the rotation of the star (up to a hundred times per second), producing the rapidly rotating, ultra-stable "light house effect" of radio, gamma ray and optical emissions that characterize the "pulsar phenomena" seen even thousands of light years away.

If a planet, like the Earth, is in line with these beams of matter, then we can "see" the lighthouse effect. If it is not, we will never spot the pulsar. In this model, because such an exotic, rapidly spinning, comparatively tiny object (but with the mass of the sun) is heavily interacting (through its now incredibly strong surface magnetic fields) with the still slowly (comparatively speaking) expanding shell of its own outer layers (from the original supernova explosion), it should also be transferring - at a measurable rate - its own finite amount of angular momentum to the larger cloud.

This has to inevitably result in a slow, steady and observable "spin down" of the neutron star.

Radio, optical and x-ray/gamma ray observations of the almost 1,000 known pulsars discovered since 1968 have measured this "spin down" effect in a wide variety of situations. The incredibly regular radio, optical and x-ray/gamma ray pulses emitted by such stars have been observed time after time to slowly lengthen by a tiny but measured amount over several years - an indication of an ultra-slow "despinning" of the tiny stars.

Using the (assumptive) law of the conservation of angular momentum, this steady spin down is viewed as confirmation not only of the known laws of angular momentum, but also as a means to "date" the ages of these stars, a kind of "pulsar clock" with a presumed constant half-life.

Because about half of all known stars are binaries, when one of these stars explodes as a Supernova it releases itself and its companion in opposite directions, at whatever the original orbital velocity was between them. In the Sagittarius pulsar, the fleeing pulsar eventually flew right out of the slowly expanding shell of gas from the original explosion (the expanding blast wave ran into an interstellar cloud and slowed way down, the neutron star core didn't).

Using the known distance, space velocity and geometry of the pulsar/ cloud relationship, the new VLA measurements of the actual space velocity of this pulsar was discovered to be only about 300 miles per second - way below the estimated 1,000 mps previously assumed.

From the observed "spin down rate," the previous estimate of the age of the neutron star/pulsar (when the original supernova exploded) was about 16,000 years-but from the "kinemetic" age of the star (measured by its known velocity beyond its own expanding envelope), the age of the original explosion is now estimated as happening about 170,000 years ago - a factor of ten disparity.

Since the now-measured space velocity of the pulsar is not open to any alternative interpretation (it's a very simple measurement, as compared to the model for a despinning pulsar), the age since the pulsar's formation (and separation from its binary companion) must be about the same: 170,000 years.

So, for 170,000 years this pulsar has been on its own, yet the rate at which its rotation is slowing indicates a much younger age. Obviously, something is radically wrong with the pulsar model of a finite amount of angular momentum slowly being expended.

The simplest explanation for this "impossibility" is that the star has been able to tap into a previously unknown source of angular momentum, which has been "trickle charging" the spin of the neutron star even as the acceleration of charged particles in its beams has been draining it at a rate which has extended the pulsar's active life approximately ten times the observed "rate" of deceleration.

Such an "unknown source" of energy is precisely predicted by the hyperdimensional model, which says that the more angular momentum an object initially possesses, the more it can "tap" into this invisible source of energy to maintain that momentum against known 3D transfer mechanisms.

The actual mechanism for maintaining the pulsar's spin is probably the conversion of the star's precessional energy (which, in DePalma's experiments, is not contingent on a nearby gravitating companion) into rotational energy. An apt analogy would be a bathtub with a hole in it.

Water is flowing out of the hole at an observed rate - but, unknown to the "observers," there is a hidden plumbing network, refilling the tub at a rate which almost, but not quite, replaces the water lost through the hole. The result is a significantly extended "lifetime" for the bathtub reservoir, but with no obvious sources of the refilling.

Result: the water in the tub drains out a lot slower than it should, even though the rate of water through the hole is well known.

There is, flatly, no other explanation for this "extra" angular momentum in pulsar B1757-24. Unlike anything that may be dreamed up by the conventional theorists in an after-the-fact attempt to patch up their broken theory, the hyperdimensional model not only implicitly - but Hoagland and Torun specifically - predicted exactly this sort of finding.

This now makes five specific predictions of Hoagland's hyperdimensional physics model, a model based on the supposedly meaningless tetrahedral alignments of the monuments of Mars that have been confirmed by empirical observations.

There is another "test bed pulsar" - PSR B1828-11 - which also seems to be on the verge of proving the hyperdimensional model right, this one via a very different set of measurements: Bruce DePalma's still-to-be-laboratory-tested hypothesis regarding "free precession."

PSR B1828-11 is an "isolated" pulsar (meaning, it is not a member of a binary star system), and is also located in the direction of Sagittarius.

In late 2000, a set of radio telescope measurements made by three Jodrell Bank astronomers revealed a remarkable property about this rapidly spinning neutron star: it had three simultaneous radio pulsar "periods," as opposed to the usual one - a "fundamental period" of about 1000 days, and three "sub-harmonics" of 500, 250 and 167 days each.

The first interpretation of this data by the discoverers was that the pulsar - despite being totally isolated - was somehow exhibiting "free space precession"; its radio beams sweeping by us on Earth at increasingly different geometries and times... in these repeating cycles... revealing the physical precession of the spinning neutron star itself!

Several alternative theoretical explanations for this remarkable behavior were immediately proposed by other astrophysicists:

"The B1828-11 pulsar, even though composed of a superdense sea of free neutrons compressed inside a sphere only about 20 kilometers across, with a surface gravity a hundred billion times the surface field of Earth, is not perfectly round; it is this slight intrinsic deformation (by less than a tenth of a millimeter) which causes PSR B1828-11 to spin slightly off-axis... to "precess."

Or:

"The pulsar's dense 'neutron sea,' flowing superconductively under its brittle surface 'crust,' hasn't quite kept pace with the solid surface's decreasing rotation (caused by the pulsar's intense magnetic braking forces); this, in turn, is causing 'procession' to occur - essentially from 'sloshing' of the lagging, internal neutron sea."

From another paper:

"The pulsar is potentially surrounded by a close-in 'accretion disc' of gas and dust, orbiting at a significant angle to the pulsar's equator. This, then, causes a hidden 'forced precessional torque,' through simple gravitational tidal effects from the orbiting material..."

And:

"PSR B1828-11 is potentially orbited by a strange matter 'quark planet' (denser than even a collapsed neutron star), which is causing the precession by its significant tidal interactions," etc., etc., etc.

Each of these theoretical attempts to explain PSR B1828-11 's bizarre behavior has serious objections - beginning with the "neutron sloshing model"; according to other astrophysical theoreticians, any internal fluid motions ("slosh" in the neutron sea) should "damp out" (dissipate energy) after only a few hundred rotations of the pulsar.

Since PSR B1828-11 is rotating two and a half times per second, and the pulsar has an estimated age of a hundred thousand years, those astrophysicists have a real problem explaining how such lagging fluid behavior could still persist... after over eight trillion neutron star rotations.

Hoagland has a very different (and simpler) explanation for this baffling behavior - drawn directly from hyperdimensional theory and DePalma's empirical experience with "rotating systems":

That PSR B1828-11 could simply be the most unambiguous Galactic evidence, so far, of actual HD precession.

In other words, a stunning astronomical example of exactly the type of laboratory "HD test" Hoagland was trying to arrange with NASA for DePalma... when DePalma died.

When asked the most fundamental rules of Hyperdimensional Physics, Hoagland will often wryly respond: "rotation ... rotation ... rotation."

Courtesy of long-time associate, David Wilcock, a few years ago Hoagland was sent several papers on an almost unknown field of Russian science. As he began reading the translations - from decades of past and current researchers, and their intensely controversial experiments in the former Soviet Union-he suddenly realized that here was a completely separate database, with literally thousands of published scientific papers, all totally consistent with DePalma's equally baffling 1970s observations of his "OD field" around rotating masses.⁴⁶

As one of these Russian reviews (written by Yu.V.Nachalov and A.N.Sokolov) noted:

"...Over the course of the XX century, various investigations in different countries, representing a variety of professional interests, repeatedly reported the discovery of unusual phenomena that could not be explained in the framework of existing theories.

 

Since these authors could not understand the physics of the observed phenomena, they were forced to give their own names to the fields, emanations and energies responsible for the creation of these phenomena.

 

For instance, N.A.Kozyrev's 'time emanation,' W.Reich's 'O-emanation' or 'orgone,' M.RBlondlot's "N-emanation,1 I.M. Shakhparonov's TVIon-emanation, A.G.Gurvich's 'mitogenetic emanation,' A.L.Chizhevsky's 'Z-emanation,' A.I.Veinik's 'chronal field,' M-field, AA.Deev's T)-field,' Yu.V.Tszyan Kanchzhen's biofield,' HMoriyama's 'X-agent,' V.V.Lensky's 'multipolar energy,' 'radiesthesietic emanation,' 'shape power,' 'empty waves,' 'pseudomagnetism,' H.A.Nieper's 'gravity field energy,' T.T.Brown's 'electrogravitation,' fifth force,' 'antigravitation,' free energy.' This list can be easily continued...."

The Russians, in this review, realized that all these apparently disparate anomalous phenomena were actually only different manifestations of the same phenomena - ultimately termed "Torsion Field Physics."

Torsion, as noted earlier, is still essentially unknown to Western science... and not by accident; until the collapse of the Soviet Union in 1991 and the sudden flood of torsion scientific literature appearing on the World Wide Web, "torsion" was literally a forbidden subject to export to the West; now, more than 20,000 research papers on torsion physics have been published in the open scientific literature - over half of them by Russian (and former Soviet Bloc) scientists.
 

Here's what one Western engineer, Paul Murad,⁴⁷ currently employed by an American institution that is researching space propulsion applications of torsion field theory has to say about the current "state-of the art":

"... The only field that (can] support faster than light phenomenon according to some Russian physicists [is] the spin or torsion field. Torsion is different from these other three fields [electrostatics, magnetics and gravities] that [hove] spherical symmetry.

 

Torsion [can] be right-handed or left-handed and is based upon a cylindrical field and can be created by large accumulations of electricity and rotation of a body that if, above a certain speed, [will] enhance the torsion field. Torsion can lead to other phenomenon to include frame dragging. Here in a vacuum, frame dragging occurs when a rod is inserted concentrically inside of a cylinder and has no physical contact with that body.

 

If the rod is suddenly removed, the cylinder will also move or is dragged along with the rod.

 

Other examples exist regarding rotational bodies that would also influence adjacent rotating bodies due to the interaction of one spin field ...with another...

"Obviously one would like to find a theory that relates all of these effects with the result of better understanding gravity. The closest thing I could find [in reading the existing Russian literature] is a comment made by Matveeko that the torsion field is identical to the transverse spin polarization of the physical vacuum and a gravitational field is identical to the longitudinal spin polarization of the physical vacuum.

 

Thus, these two fields, gravity and torsion, appear to be related and may be the key [relationship we must understand] before we learn how to harvest [limitless] energy fromthe physical vacuum or the zero point field.

 

These issues are all interesting theories and definitely should be further explored if mankind-wishes to get serious about space travel to planets and the far horizons."

The theoretical "father" of torsion physics is generally considered to be French mathematician, Dr Elie-Joseph Cartan, who in 1913 published a refinement of Einstein's General Theory of Relativity, whereby curved "spacetime" could flow in spiral patterns around rotating objects, a phenomenon not originally dealt with in Relativity, termed "torsion."

Ultimately known as "Einstein-Cartan Torsion (ECT)," the initial physical predictions were extremely limiting and disappointing; the resulting forces from ECT were calculated to be some "27 orders of magnitude (27 powers of ten!) smaller than the gravitational effects of Relativity."

Further, these effects were calculated to be restricted to static (non-moving) field geometries around rotating objects, fields that could not propagate through space as "waves."

Because of these severe limitations, ECT is thought by most physicists (those who even know about Cartan's contributions to Relativity theory) to be, at best, a minor curiosity - and to play an almost infinitesimal role in the universe at large, even at the sub-atomic level.

However, later Russian theoreticians (like Dr. Gannady Shipov),⁴⁸ applying separate torsion ideas originally propounded by the 17^th-century philosopher, Rene Descartes - that, ultimately all motion (even apparently linear motion) is "rotation" (in a "curved" universe) - would go on to prove that torsion fields are ultimately not static (as Cartan had calculated from his erroneous assumptions regarding what constitutes "rotation"), but actually dynamic.

Dynamic torsion (also called "Ricci torsion" - after the ^^-century Italian mathematician who refined Decartes ideas and combined them with the pre-Relativity spatial geometry of Bernhard Riemann) is generated by any moving and simultaneously rotating object [from spinning atoms, to entire nlanets (especially those precessing); from orbiting stars, to entire galaxies stars ...].

The calculated strength of dynamic torsion fields is something like "21 to 22 orders of magnitude stronger" than Cartan's "static fields."

Not only that, but these fields can travel... as a "torsion wave" through spacetime - capable (in the equations of some Russian theorists cited by Murad - above) of exceeding the vacuum speed of light by at least a billion times.

(That's the lower bound, the actual speed could be a lot higher; the theoretical maximum velocity at which a dynamic torsion wave can travel is actually still quite unknown - Fig. 2-11...)

For those having trouble visualizing how "torsion" works, how it compares to more familiar forms of information and energy transmission - such as electromagnetic radiation - perhaps a couple of analogies will help; if spacetime (Maxwell's "ether") is pictured as "a 2D porous, geometric structure" - like a very thin sponge, or maybe a paper towel - then electromagnetic energy can be pictured as water seeping through the sponge or towel at a finite rate of speed (as a substitute, in our analogy, for "C" - the "velocity of light in a vacuum").

Now, in this thought experiment, allow a drop of water to fall on the towel/sponge, entering its 2D surface (and bringing in additional energy ...) from "a higher dimension." Two things will simultaneously occur:

On impact, the droplet will create ripples in the water in the towel or sponge (remember, our fluid analog to electromagnetic radiation), similar to raindrops on a pond [Fig. 2-10]; simultaneously, the impact will also create invisible sound waves within the material structure of the towel/sponge (our analogy with the geometric structure of our 3D ether).

Since the speed of sound in this material structure is much faster than the speed of pressure waves (ripples) in the water... the information regarding the input of new energy into the sponge/towel structure, arriving from a "higher dimension," will be communicated almost instantly throughout that structure via the sound waves its appearance triggers... while the tiny water ripples, set up by the same impact, will take much longer to physically flow to every Portion of the towel/sponge...

In our analogy, this difference in relative speeds represents the vast gap in velocity between electromagnetic radiation - limited to "the speed of light" in our 3D reality - and dynamic torsion, which (according to Kozyrev's own astronomical measurements) can travel - as a spiraling wave - through the ether incomparably faster...

The reality of "torsion physics" - information communicated through the ether from a higher dimension, analogous to invisible and much faster sound waves, compared to "physical ripples on a pond" [Fig. 2-10] - changes everything.

Suddenly, the bizarre "OD field effects" observed by Depalma surrounding his rotating gyroscopes, able to somehow influence the spin of other rotating objects even in distant rooms, and the equally mysterious "non-Newtonian pendulum anomalies," discovered by Nobel Laureate Dr. Maurice Allais during a total solar eclipse over Paris, in 1954, all are suddenly revealed to stem from an identical foundation-Modifications to Einstein's fundamental "Theory of General Relativity."

If Einstein and Cartan are the "god fathers" of current torsion theory, the late Russian astronomer - Dr Nikolai A. Kozyrev - is definitely the "building architect" of this new science.

Kozyrev, a Soviet astrophysicist, became world-famous in 1958 for his controversial spectroscopic detection of the first apparent gas emissions from the Moon (thus indicating it was, at some level, still active geologically).

In parallel to his major astronomical career, Kozyrev also quietly conducted 33 years of empirical laboratory investigations of "rotation on rotation" behind the Iron Curtain.49 This work was completely independent of DePalma's eerily similar, equally painstaking efforts in the West.

Revealing the astronomical entry point for his "new physics," Kozyrev wrote in 1963:

"... It is of interest that even such a concrete question - namely, why do the Sun and the stars shine, i.e., why are they out of thermal equilibrium with the ambient space - cannot be answered within the known physical laws...."

In the end, all these scientists - DePalma, Kozyrev and Hoagland, separated by half a world and two completely different ideologies - independently confirmed the same inexplicable phenomena surrounding "rotation," and the simultaneous appearance of anomalous energy into all rotating objects as a result... an energy somehow, in Kozyrev's prophetic words, coming from beyond "the known physical laws."

The difference is Kozyrev's 33 years of countless laboratory demonstrations of this physics (and consistently anomalous results) eventually inspired a new generation of Russian mathematical physicists - like Shipov, decades later - to search out the theoretical foundations of these multiple "torsion phenomena."
 

It is safe to say that, without the major work of Nikolai Kozyrev, the currently exploding field of "torsion physics" - based on decades of his repeatable experiments - simply would not have occurred.

And, without Hoagland's serendipitous discovery of Kozyrev's work in 2005, "hyperdimensional physics" would still be lacking the sweeping experimental and mathematical foundation of "torsion physics" it has now suddenly discovered is its direct heritage.

For, surprise, surprise...

The energy and information existing in higher physical dimensions, accessible in three dimensions only through the physical "rotation" of mass, is the ultimate source of all "torsional phenomena" that Kozyrev observed...

In 1993, the Angstrom Foundation, in Stockholm, Sweden, awarded the "International Angstrom Medal for Excellence in Science" to Hoagland for his role in rediscovering the hyperdimensional physics upon which Maxwell's original treatises were built.

You might think, based on all the information just outlined, that the theory of "hyperdimensional physics" - replete with correlated observations, testable predictions and significant experimental successes - should be making quite a bit of noise in the world of advanced theoretical physics. Regardless of the reductionist arguments, Hoagland and Torun formed a coherent, productive and eminently testable model of the reality of the Cydonia artifacts.

This model contains no less than eight specific, testable predictions, five of which have already been confirmed or have been supported by initial observations. By any reasonable standard, that would be more than sufficient (one would think) for conventional science to at least consider taking the ideas and their source (Cydonia) seriously.

Instead, with the exception of the Angstrom Foundation, the political reaction has been stone silence.

Hoagland, who at one time was warmly embraced by various NASA facilities and programs, suddenly found himself on the outs with those same institutions when he pressed the issue of the tetrahedral mathematics of Cydonia.

His ideas were welcomed, it seemed, as long as there was no real means of proving his hypothesis. It was only when he ventured into the realm of hyperdimensional physics and sought for it the same status as any other testable theory that NASA suddenly decided it would no longer lend an ear to his ideas.

It was at this point - as we entered the 1990s - that we began to suspect that there was something seriously wrong with this picture.

Chapter Two Images

 

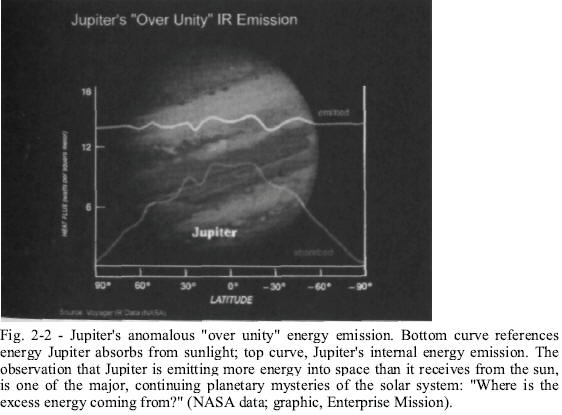
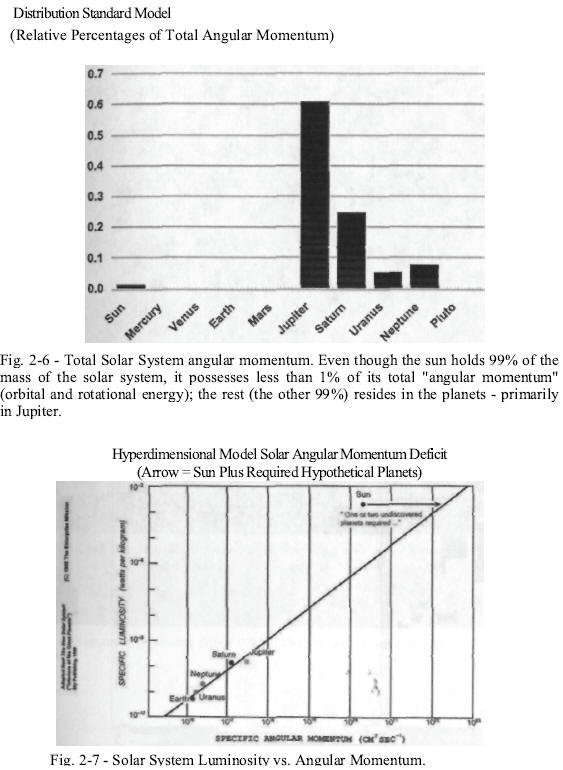
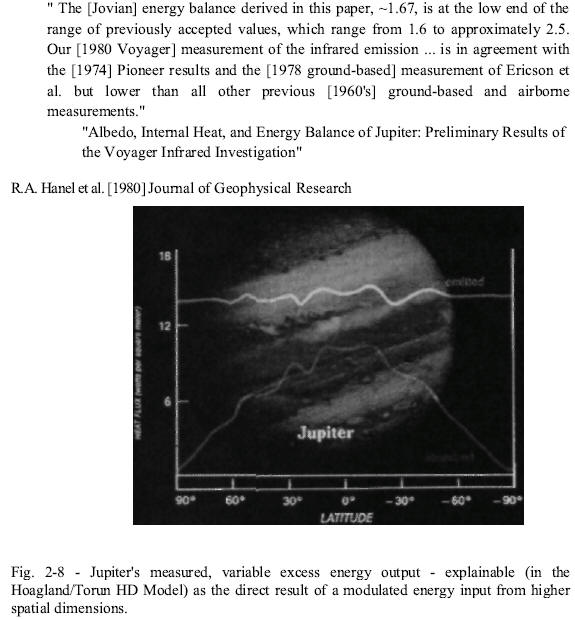
Back to Contents