|
from
Medium Website
Its structure is the culmination of 4 billion years of improbable development, beginning with simple biomolecules and exploding into a dynamic, hyperconnected network whose complexity we're still trying to decipher today.
And it's not only physically complex, but is crucially and inexplicably linked to something beyond its material constitution:
This - the age-old
paradox of the mind-body problem - elevates the brain from the
level of inert matter into something that demands more mystery and
intrigue.
These are black holes and wormholes:
Spoiler alert here... but who can forget when the protagonist of Interstellar interacts with the past by entering a black hole, or when the cyber-prisoners of the Black Mirror episode USS Calister escape through a software update disguised as a wormhole?
Our public imagination
reflects fascination and wonder at these objects.
By contrast, there's a
special class of astrophysical concepts that simply go beyond these
categories. This is intriguing from a metaphysical perspective,
since we consider these categories as fundamentally constitutive of
reality.
The story begins with one of Einstein's favorite subjects:
Gravity - Force or
Curvature?
Given two particles m1 and m2 and their distance r, the gravitational force between them can be given by the equation,
Here, G represents the
gravitational constant, an experimentally-derived number which keeps
the dimensions of the equation consistent.
This was a significant statement at the time, since the mechanics of celestial bodies was still being attributed to some mysterious 'ether', or quintessence, whose physical dynamics was considered separate to that of Earth.
With Newton, however, the
entire cosmos was now in the grasp of known physics, and,
importantly, within the predictive capacities of mankind.
Pre-Newtonian physics situated the celestial objects
in a ring of aether
The error margin associated with Newton's equation for gravity is imperceptible for most applications here on Earth, but not elsewhere.
In the late 19th century physicists were already aware that the expected orbit of Mercury given Newton's equations deviated slightly - but significantly - from observation.
At the time, this was
considered a temporary problem whose solution would be some error in
measurement. It was difficult to doubt a concept which had such a
lasting impact on European science and philosophy.
In 1915, Einstein developed a set of 10 field equations which described his new theory of general relativity.
Plugging mass into these equations allows for a calculation of gravity which models Mercury's orbit in perfect agreement with measurements. Einstein's equations also predicated that light, a massless particle, should be curved by gravity, an idea which was totally antithetical to Newton's framework.
But this prediction, along with literally hundreds of others, were later confirmed by experiments.
Einstein's theory has stood the test of time:
The difference, however, between Einstein's theory of relativity and Newton's conception of gravity is more than one of numerical accuracy and predictive power.
There is a fundamental conceptual antagonism between the two, rooted in a disagreement on the philosophical categories of space and time themselves.
There appears to be a static, absolute 'grid' of space, along with an extra coordinate of time ticking steadily in the background. Gravity, in that sense, is simply a force that pulls objects along out there in the space-and-time arena of the world.
If we wanted to describe
the path a cannonball takes while traveling in the air, it's enough
to just draw a Cartesian grid labeling space and time, and treat
gravity like a force that moves objects along the coordinate system.
Instead, gravity itself is a warping of space and time, whose effects on objects we mistake as a force.
And crucially, space and time are not 'warped' separately, but on a single manifold known as spacetime:
Thus, instead of a force,
an object's acceleration due to gravity is simply its straight-line
path (or geodesic) in a patch of spacetime that is curved by the
presence of some mass.
That's a tricky question, but one thing is true:
In spacetime, for example, events that I observe to happen at the same time can happen at different times in another person's perspective.
This apparent paradox, known as the relativity of simultaneity, is not allowed by our intuitive understanding of space and time. In addition, clocks tick slower when they are closer to a massive body due to the influence of gravitational time dilation.
These kinds of examples,
many of which have been demonstrated experimentally, illustrate that
Newton's philosophical understanding of space and time - intuitive
as they are - must be invalid.
Photons are massless particles, so classical physics wouldn't predict that gravity interacts with them. However, if gravity is understood as curvature, then even light should be 'bent' by the presence of a massive object since it too has a path in spacetime.
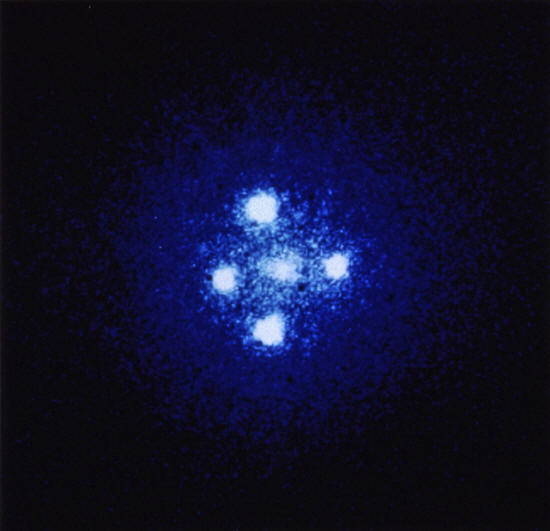
This finding is called gravitational lensing, and has been demonstrated by observing a a number of cosmological oddities. One of these is Einstein cross, a quasar that is quadrupled by the presence of another galaxy.
These kite-shaped lights
come from a single source, whose gravitational effects make it
appear like 4 objects from our perspective.
Einstein cross
To demonstrate this, we have to understand that Einstein's equations for gravity can be used to model the entire universe.
Because gravity is the predominant force guiding the interaction of these clusters, Einstein's field equations can be used to model the evolution of the universe over time.
All physicists need to do
is use data collected by telescopes and 'plug in' the corresponding
values of mass to simulate the past and future conditions of our
universe.
Edwin Hubble provided experimental evidence that the universe is expanding by observing the radiation emitted from distant galaxies. This fact can be modeled into the Einstein field equations, resulting in an expanding spacetime manifold.
Reversing time in this
system leads us to a singular point of infinite density and
temperature, called
the singularity. All events which occur in our
universe necessarily come after this point, leading to its popular
characterization as the as the 'origin point' of our universe.
The 'orientation' of spacetime in this region would be such that any light entering a certain region, called the event horizon, can never exit it again. To be more precise, the fabric of spacetime is contorted in such a way that the spatial direction towards a certain point becomes the temporal direction of the future.
This shuttles whatever
enters a certain parameter into a necessary future, 'located' at a
central part called a singularity, which is the end-point of the
future-radial path.
But let's try to understand the popular phrase:
Within a certain region of space demarcated by the event horizon, not only objects or light, but events themselves are forever shut-off from us.
Another way to put it is this:
This is what causes black holes to appear black to us.
It isn't because light is being 'sucked in' by some invincible force:
This is the kind of
thinking one has to grapple with when speaking about a single
spacetime manifold.
If the Einstein field equations can be used to model our universe with stunning accuracy, then does everything the equation produce need to have a correlate in our reality?
As many of us remember, quadratic equations can be used to model the path of a projectile. But solving these equations also gives us a negative value for the horizontal displacement, -x.
Even weirder, this would involve a negative time value.
It's clear in this example that we shouldn't take our math too seriously.
Physicists were in a
similar position: did the black hole solutions to Einstein's field
equations necessarily describe a real feature of our universe, or
were they merely mathematical objects?
The resulting structure is called an accretion disk:
The electromagnetic radiation emitted by this disc eventually reached an X-ray detector here on Earth, and analyzing the data revealed the central object's mass density to be consistent with that of a black hole.
This explanation was enough for big-daddy of cosmology Stephen Hawking to concede a bet to a colleague about the existence of black holes in 1990.
Today, they're one of the
most well-known objects in astronomy.
Objects are always emitted from a single point, so we can see nothing but light emanate from it; from the inside of the event horizon, the only future is radially outward.
These objects are appropriately named white holes.
The question of whether
white holes exist or are just mathematical oddities hasn't quite
been settled. The gamma ray burst GRB 060614 is considered a white
hole candidate by some, owing to its rule-breaking properties.
As I mentioned earlier, it's all a matter of mass density. The Schwartzschild radius of a massive object gives the minimum space it needs to occupy to become a black hole.
For example, if the Sun's mass was compressed into a sphere with a radius of 3.0 kilometers, its density would cause it to collapse into a singularity, and all the 'space' within the resulting event horizon would fall outside of our causal universe.
However, there's nothing
particularly 'special' about the way that black holes effect the
universe outside of their event horizon. If the Sun were to collapse
into a black hole, our gravitational orbit around the singularity
would be essentially unchanged.
In this way, stars and nebulae are effectively physically erased from our universe. However, this presents a contradiction with quantum physics, whose equations require that information can never be destroyed.
This problem, called the black hole information paradox, suggests that a new theory must be used to combine the worlds of general relativity and quantum mechanics.
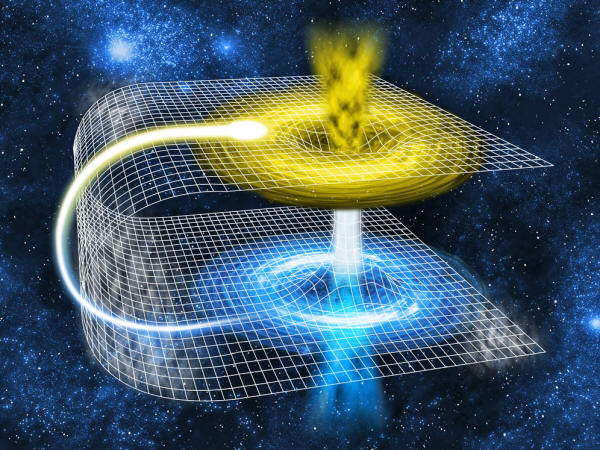
Along with the infamous warp-drive, traversing a wormhole is among the most common methods for intergalactic travel.
As the story goes,
wormholes connect two points of spacetime in such a way that
physically traveling between them becomes unnecessary. Thus, one can
'jump' to a certain point in the universe without being hindered by
that cosmic limit, the speed of light.
Because of this, when the black hole is modeled, the white hole appears to be spitting out its contents into 'another universe' which is causally separated from the first.
This means that, mathematically speaking,
The details of this
structure were published in 1935, and came to be known as
Einstein-Rosen bridges after the authors of the paper.
Einstein himself developed these structures in an ill-fated attempt to combine his theory of general relativity with electrodynamics.
For that reason, the 'throat' of the bridge was initially only big enough to carry an electron. Then, in 1964, physicist John Archibald Wheeler proved that these types of wormholes are unstable and would collapse before a single photon would be able to traverse it.
This came as a relief to
some physicists, as the idea of faster-than-light travel causes
problems with our ideas of causality.
Kip Thorne has argued that an exotic kind of matter, one with a negative energy density, would be sufficient to keep the wormhole open for a courageous enough traveler.
These are known as traversable wormholes, and once they are created, the two ends would begin in the same place and would have to be physically separated. This is not such a grave limitation, however, since we could keep sending new wormholes through the distance created by the previous set.
In this way, our universe
may someday be riddled with these gateways, emanating like roadways
from their central origin here in the solar system.
Interestingly, the vacuum of space itself seems to be a good candidate. In a finding called the Casimir effect, two plates held a microscopic distance apart can either attract or repel slightly, depending on their orientation.
But this is not due to gravitational force, or any other kind of standard force for that matter: rather, it is due to the intrinsic qualities of empty space.
The quantum mechanics
underlying the Casimir effect is complicated, but it demonstrates
that the type of negative energy necessary for propping upon a
wormhole may already exist naturally.
This accounts for their popular depiction as a bridge between the two sides of a folded paper.
This isn't as inaccurate a description as we might think, as long as we wrap our heads around the idea that what's folding is not a physical surface, but spacetime itself...
In other words, what's
folding is a field that gives us space and time coordinates when we
measure them; we really can't say much about the actual ontology of
spacetime.
The course of scientific progress and development will gives us the answer, but it's probably not discernible within our lifetimes.
Using the tools of strict
empiricism and mathematics, we've been able to arrive at some
strange but compelling conclusions about the nature of space, time,
causality, and so on - topics that were previously the exclusive
domain of natural philosophy.
It's enough for us to stop and ask:
The difficulties of these
questions are a fresh reminder that, when it comes to knowledge of
the Universe, we are still infants in a vast sea of the unknown...
|